Bhagya

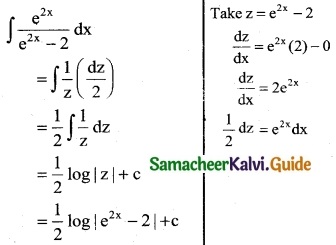
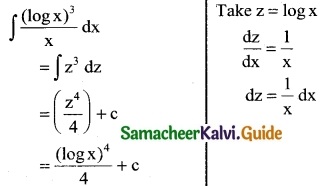
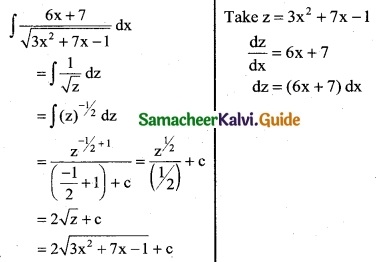
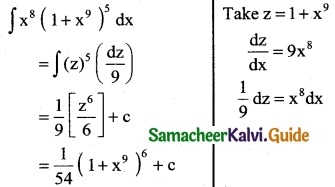
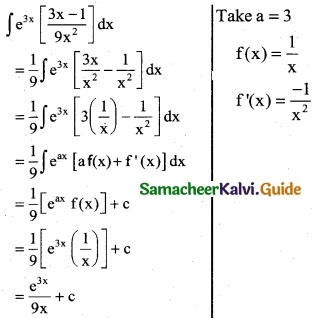
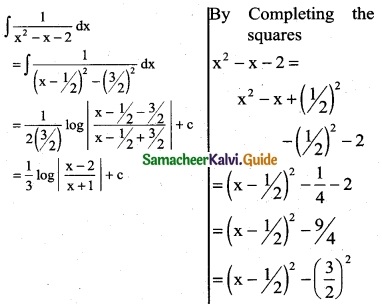
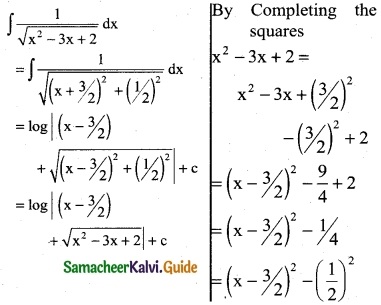
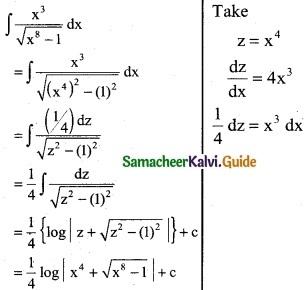
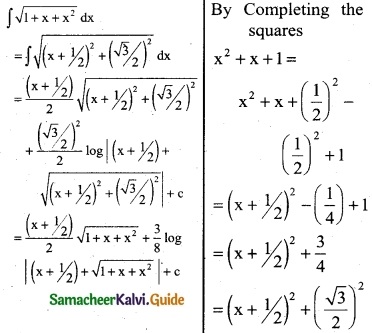
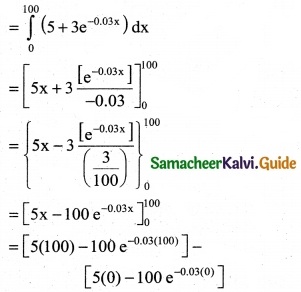
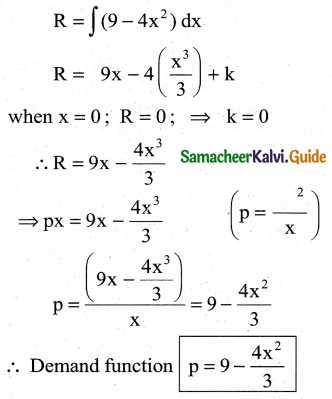
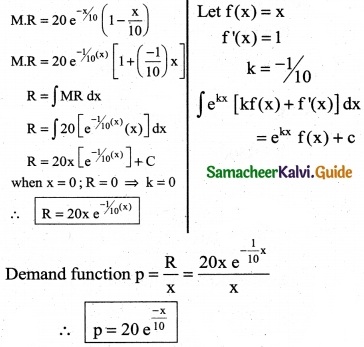
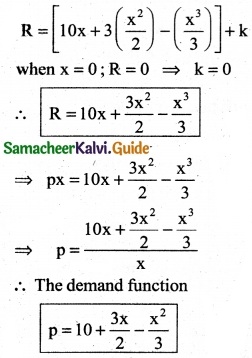
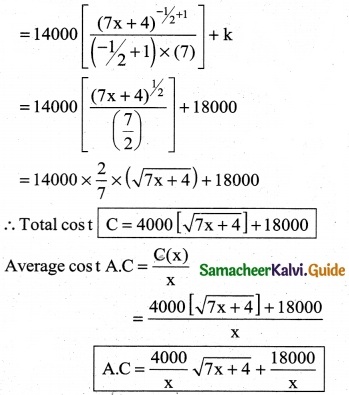
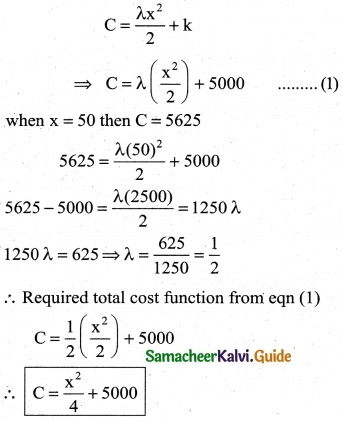
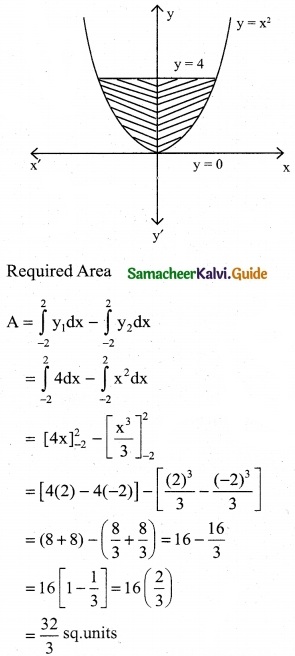
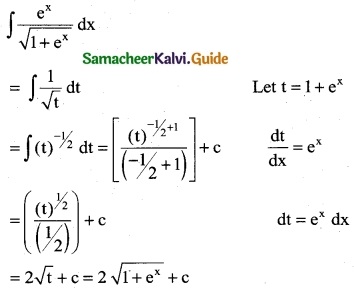
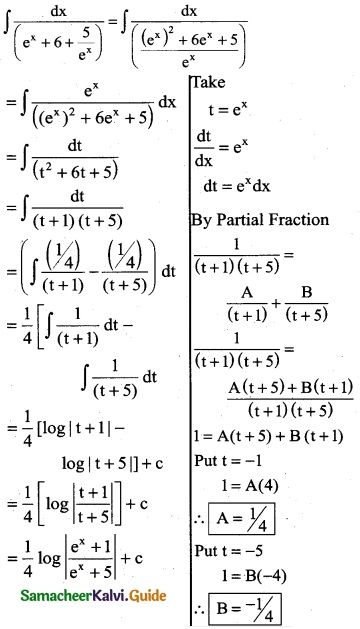
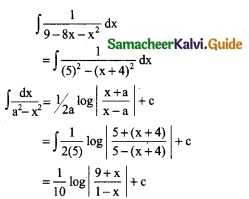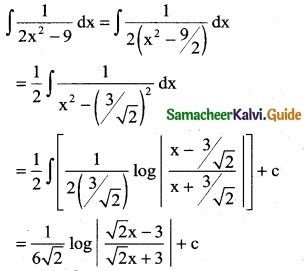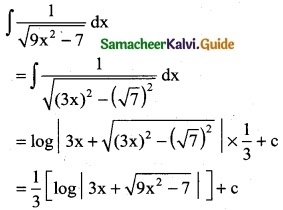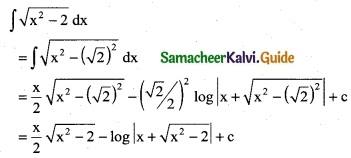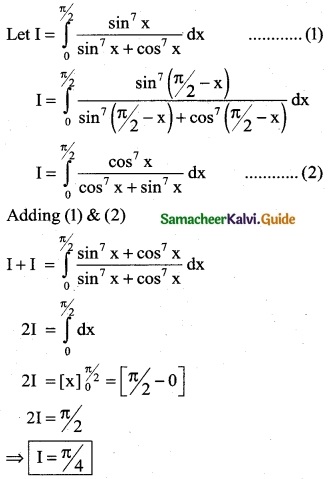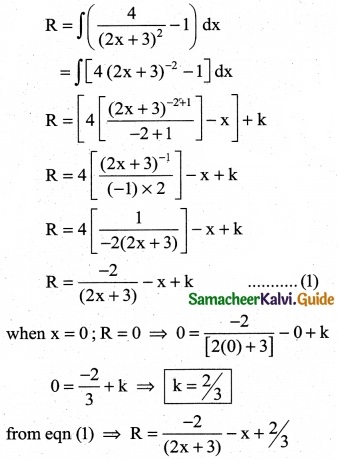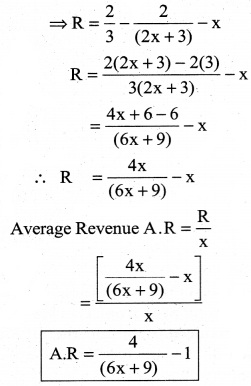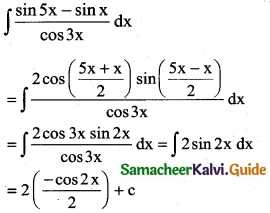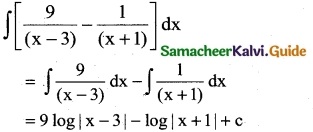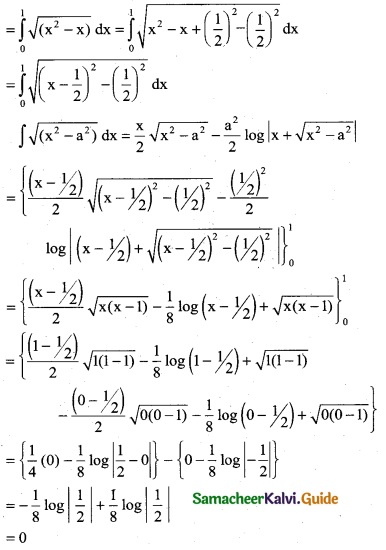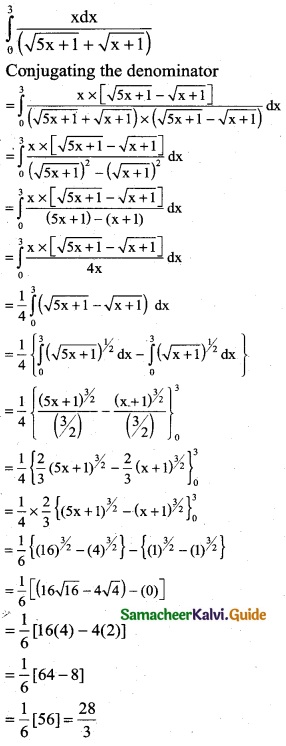Students can download Maths Chapter 6 Trigonometry Ex 6.4 Questions and Answers, Notes, Samacheer Kalvi 10th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 10th Maths Solutions Chapter 6 Trigonometry Ex 6.4

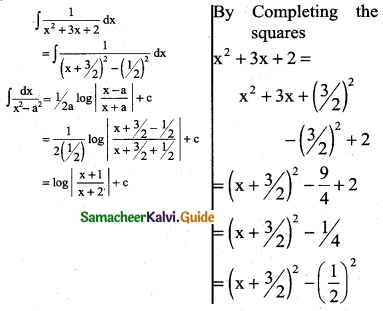
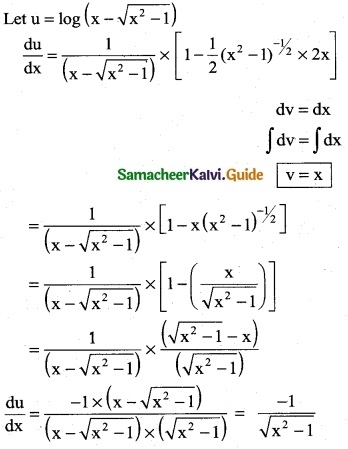
Question 1.
From the top of a tree of height 13 m the angle of elevation and depression of the top and bottom of another tree are 45° and 30° respectively. Find the height of the second tree, (\(\sqrt { 3 }\) = 1.732)
Answer:
Let the height of the second tree be “h”
ED = (h – 13) m
Let AB = x m
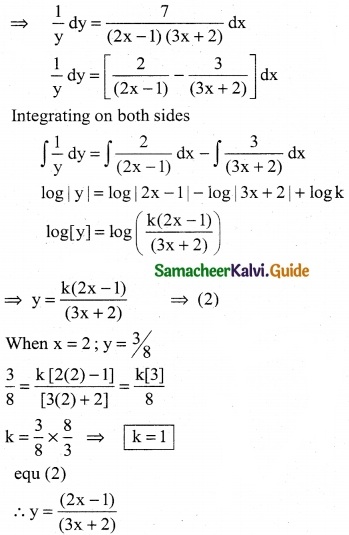
In the right ∆ ABC, tan 30° = \(\frac { BC }{ AB } \)
\(\frac{1}{\sqrt{3}}\) = \(\frac { 13 }{ x } \)
x = 13 \(\sqrt { 3 }\) ……..(1)

In the right ∆ CED, tan 45° = \(\frac { DE }{ EC } \)
1 = \(\frac { h-13 }{ x } \)
x = h – 13 ……..(2)
From (1) and (2) we get
h – 13 = 13 \(\sqrt { 3 }\) ⇒ h = 13 \(\sqrt { 3 }\) + 13
= 13 × 1.732 + 13
= 22.52 + 13 = 35.52 m
∴ Height of the second tree = 35.52 m

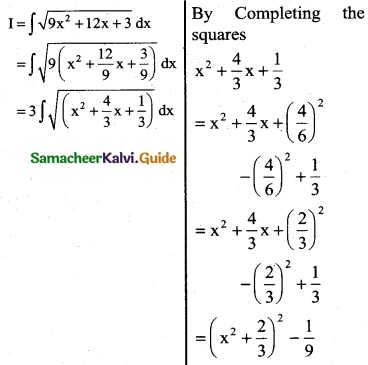
Question 2.
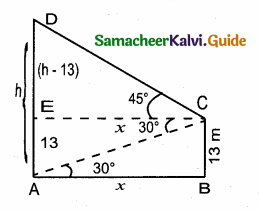
A man is standing on the deck of a ship, which is 40 m above water level. He observes the angle of elevation of the top of a hill as 60° and the angle of depression of the base of the hill as 30° . Calculate the distance of the hill from the ship and the height of the hill. (\(\sqrt { 3 }\) = 1.732)
Answer:
Let the height of the hill BE be “h” m and the distance of the hill from the ship be “x” m
In the right ∆ ABD
tan 30° = \(\frac { AD }{ DB } \)
\(\frac{1}{\sqrt{3}}=\frac{40}{x}\)
x = 40 \(\sqrt { 3 }\) ……..(1)
In the right ∆ CDE
tan 60° = \(\frac { CE }{ DC } \)
\(\sqrt { 3 }\) = \(\frac { h-40 }{ x } \)
x = \(\frac{h-40}{\sqrt{3}}\) ……..(2)

From (1) and (2) we get
\(\frac{h-40}{\sqrt{3}}\) = 40\(\sqrt { 3 }\)
h – 40 = 40 × 3
h = 120 + 40 = 160 m
Height of the hill = 160 m
Distance of the hill from the ship = 40 × \(\sqrt { 3 }\) = 40 × 1.732 = 69.28 m

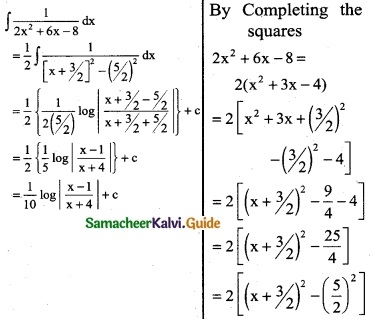
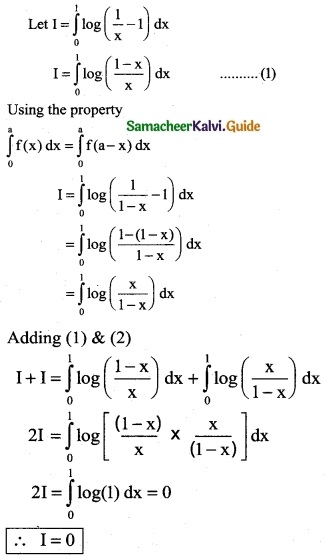
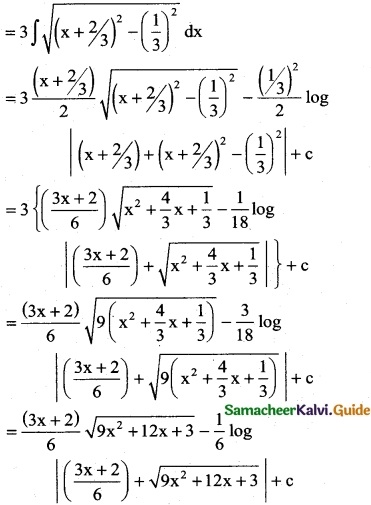
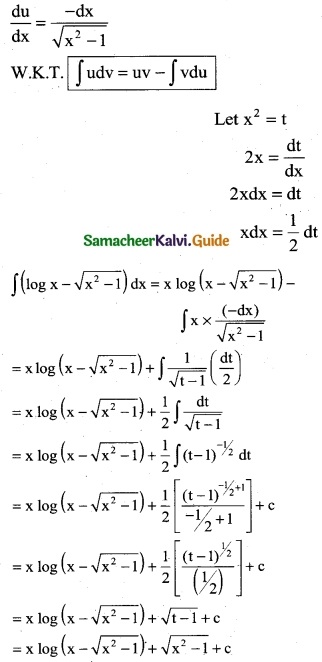
Question 3.
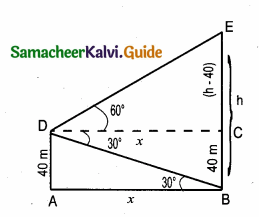
If the angle of elevation of a cloud from a point ‘h’ metres above a lake is θ1 and the angle of depression of its reflection in the lake is θ2. Prove that the height that the cloud is located from the ground is \(\frac{h\left(\tan \theta_{1}+\tan \theta_{2}\right)}{\tan \theta_{2}-\tan \theta_{1}}\)
Answer:
Let P be the cloud and Q be its reflection.
Let A be the point of observation such that AB = h
Let the height of the cloud be x. (PS = x)
PR = x – h and QR = x + h

Let AR = y
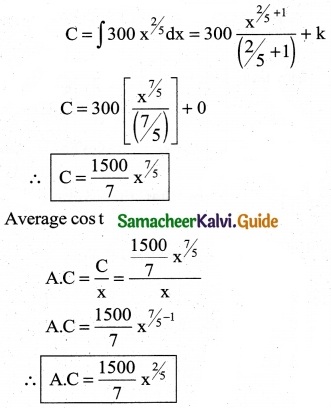
In the right ∆ ARP, tan θ₁ = \(\frac { PR }{ AR } \)
tan θ₁ = \(\frac { x-h }{ y } \) ………(1)
In the ∆ AQR,
tan θ₂ = \(\frac { QR }{ AR } \)
tan θ₂ = \(\frac { x+h }{ y } \) ……….(2)
Add (1) and (2)

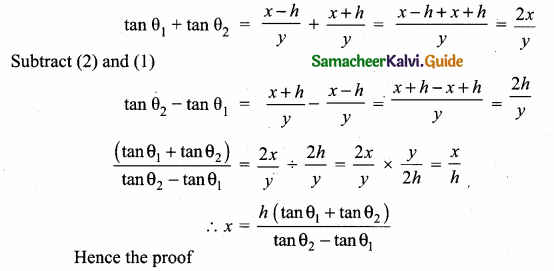
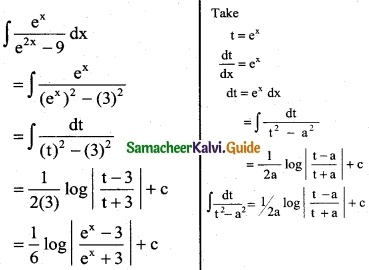
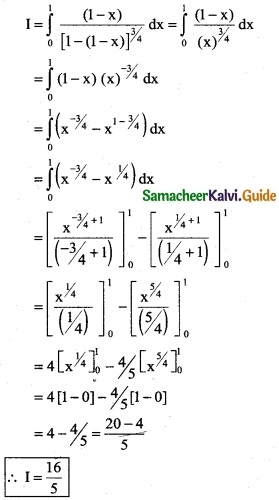
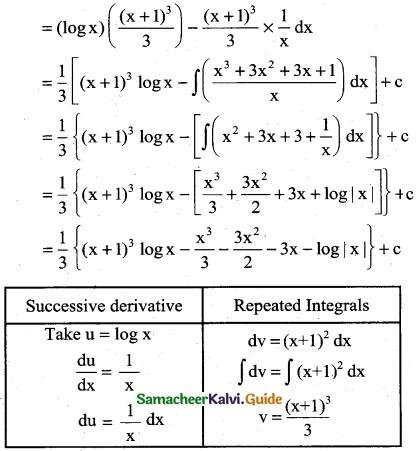
Question 4.
The angle of elevation of the top of a cell phone tower from the foot of a high apartment is 60° and the angle of depression of the foot of the tower from the top of the apartment is 30° . If the height of the apartment is 50 m, find the height of the cell phone tower. According to radiations control norms, the minimum height of a cell phone tower should be 120 m. State if the height of the above mentioned cell phone tower meets the radiation norms.
Answer:
Let the height of the cell phone tower be “h” m
AD is the height of the apartment; AD = 50 m
Let AB be “x”
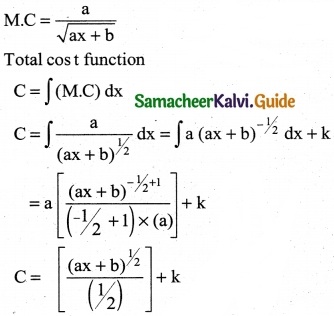
In the right triangle ABC
tan 60° = \(\frac { BC }{ AB } \)
\(\sqrt { 3 }\) = \(\frac { h }{ x } \)

x = \(\frac{h}{\sqrt{3}}\) …….(1)
In the right triangle ABD, tan 30° = \(\frac { AD }{ AB } \)
\(\frac{1}{\sqrt{3}}\) = \(\frac { 50 }{ x } \)
x = 50 \(\sqrt { 3 }\) ……(2)
From (1) and (2) We get
\(\frac{h}{\sqrt{3}}\) = 50 \(\sqrt { 3 }\)
h = 50\(\sqrt { 3 }\) × \(\sqrt { 3 }\) = 50 × 3 = 150
Height of the cell phone tower is 150 m.
Yes, the cell phone tower meets the radiation norms.

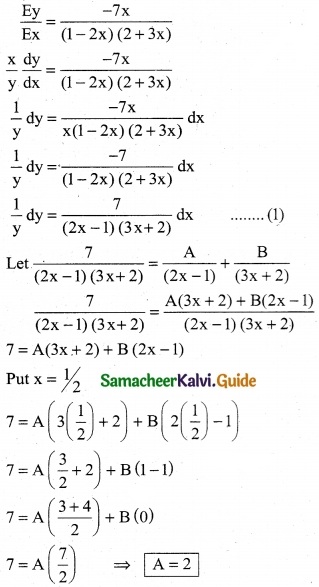
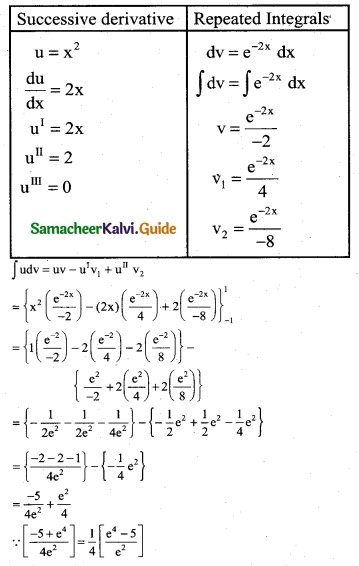
Question 5.
The angles of elevation and depression of the top and bottom of a lamp post from the top of a 66 m high apartment are 60° and 30° respectively. Find
(i) The height of the lamp post.
(ii) The difference between height of the lamp post and the apartment.
(iii) The distance between the lamp post and the apartment. (\(\sqrt { 3 }\) = 1.732)
Answer:
(i) Let the height of the lamp post AE be “h” m
DE = h – 66
Let AB be “x”
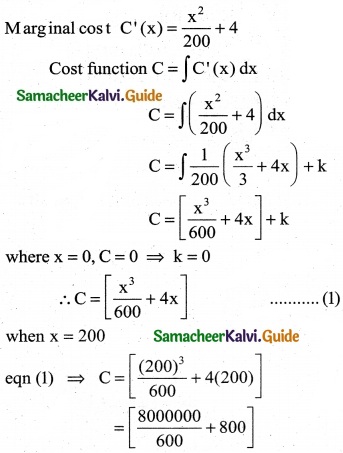
In the right ∆ ABC, tan 30° = \(\frac { BC }{ AB } \)
\(\frac{1}{\sqrt{3}}=\frac{66}{x}\)
x = 66 \(\sqrt { 3 }\) ……(1)

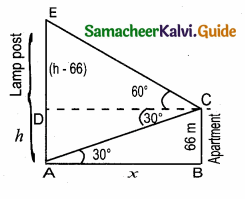
In the right ∆ CDE, tan 60° = \(\frac { DE }{ DC } \)
\(\sqrt { 3 }\) = \(\frac { h-66 }{ x } \) ⇒ \(\sqrt { 3 }\) x = h – 66
x = \(\frac{h-66}{\sqrt{3}}\) ………….(2)
From (1) and (2) we get
\(\frac{h-66}{\sqrt{3}}\) = 66 \(\sqrt { 3 }\)
h – 66 = 66 \(\sqrt { 3 }\) × \(\sqrt { 3 }\) = 66 × 3
h – 66 = 198 ⇒ h = 198 + 66
h = 264 m
(i) the height of the lamp post = 264 m
(ii) Difference of the height of lamp post and apartment = 264 – 66
= 198 m
(ii) Distance between the lamp post and the apartment = 66 \(\sqrt { 3 }\) m
= 66 × 1.732 = 114.31 m

Question 6.
Three villagers A, B and C can see each other across a valley. The horizontal distance between A and B is 8 km and the horizontal distance between B and C is 12 km. The angle of depression of B from A is 20° and the angle of elevation of C from B is 30°. Calculate:
(i) the vertical height between A and B.
(ii) the vertical height between B and C. (tan 20° = 0 .3640, \(\sqrt { 3 }\) = 1. 732)
Answer:
Let AD is the vertical height between A and B
In the right ∆ ABD

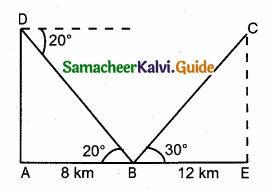
tan 20° = \(\frac { AD }{ BD } \)
0.3640 = \(\frac { AD }{ 8 } \)
AD = 0.3640 × 8 = 2.912 km
∴ AD = 2.91 km
CE is the vertical height between C and B
In the right ∆ BCE, tan 30° = \(\frac { CE }{ BE } \)
\(\frac{1}{\sqrt{3}}=\frac{C E}{12} \Rightarrow \sqrt{3} C E=12\)
CE = \(\frac{12}{\sqrt{3}}=\frac{12 \times \sqrt{3}}{\sqrt{3} \times \sqrt{3}}=\frac{12 \times \sqrt{3}}{3}\)
= 4 \(\sqrt { 3 }\) = 4 × 1.732 = 6.928
= 6.93 km
(i) The vertical height between A and B = 2.91 km
(ii) The vertical height between B and C = 6.93 km

Read More:

INDIACEM Pivot Point Calculator

Students can download Maths Chapter 2 Relations and Functions Unit Exercise 2 Questions and Answers, Notes, Samacheer Kalvi 10th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.

Tamilnadu Samacheer Kalvi 10th Maths Solutions Chapter 2 Numbers and Sequences Unit Exercise 2

Question 1.
Prove that n² – n divisible by 2 for every positive integer n.
Answer:
We know that any positive integer is of the form 2q or 2q + 1 for some integer q.
Case 1: When n = 2 q
n² – n = (2q)² – 2q = 4q² – 2q
= 2q (2q – 1)
In n² – n = 2r
2r = 2q(2q – 1)
r = q(2q + 1)
n² – n is divisible by 2

Case 2: When n = 2q + 1
n² – n = (2q + 1)² – (2q + 1)
= 4q² + 1 + 4q – 2q – 1 = 4q² + 2q
= 2q (2q + 1)
If n² – n = 2r
r = q (2q + 1)
∴ n² – n is divisible by 2 for every positive integer “n”

Question 2.
A milk man has 175 litres of cow’s milk and 105 litres of buffalow’s milk. He wishes to sell the milk by filling the two types of milk in cans of equal capacity. Calculate the following
(i) Capacity of a can
(ii) Number of cans of cow’s milk
(iii) Number of cans of buffalow’s milk.
Answer:
175 litres of cow’s milk.
105 litres of goat’s milk.
H.C.F of 175 & 105 by using Euclid’s division algorithm.
175 = 105 × 1 + 70, the remainder 70 ≠ 0

Again using division algorithm,

105 = 70 × 1 + 35, the remainder 35 ≠ 0
Again using division algorithm.
70 = 35 × 2 + 0, the remainder is 0.
∴ 35 is the H.C.F of 175 & 105.
(i) ∴ The milk man’s milk can’s capacity is 35 litres.
(ii) No. of cow’s milk obtained = \(\frac { 175 }{ 35 } \) = 5 cans
(iii) No. of buffalow’s milk obtained = \(\frac { 105 }{ 35 } \) = 3 cans

Question 3.
When the positive integers a, b and c are divided by 13 the respective remainders are 9,7 and 10. Find the remainder when a + 2b + 3c is divided by 13.
Answer:
Given the positive integer are a, b and c
a = 13q + 9 (divided by 13 leaves remainder 9)
b = 13q + 7
c = 13q + 10
a + 2b + 3c = 13q + 9 + 2(13q + 7) + 3 (13q + 10)
= 13q + 9 + 26q + 14 + 39q + 30
= 78q + 53
When compare with a = 3q + r
= (13 × 6) q + 53
The remainder is 53

Question 4.
Show that 107 is of the form 4q +3 for any integer q.
Solution:
107 = 4 × 26 + 3. This is of the form a = bq + r.
Hence it is proved.

Question 5.
If (m + 1)^th term of an A.P. is twice the (n + 1)^th term, then prove that (3m + 1)^th term is twice the (m + n + 1)^th term.
Answer:
t_n = a + (n – 1)d
Given t_m+1 = 2 t_n+1
a + (m + 1 – 1)d = 2[a + (n + 1 – 1)d]
a + md = 2(a + nd) ⇒ a + md =2a + 2nd
md – 2nd = a
d(m – 2n) = a ….(1)
To Prove t_{(3m + 1)} = 2(t_m+n+1)
L.H.S. = t_3m+1
= a + (3m + 1 – 1)d
= a + 3md
= d(m – 2n) + 3md (from 1)
= md – 2nd + 3md
= 4md – 2nd
= 2d (2m – n)
R.H.S. = 2(t_m+n+1)
= 2 [a + (m + n + 1 – 1) d]
= 2 [a + (m + n)d]
= 2 [d (m – 2n) + md + nd)] (from 1)
= 2 [dm – 2nd + md + nd]
= 2 [2 md – nd]
= 2d (2m – n)
R.H.S = L.H.S
∴ t_(3m+1) = 2 t_(m+n+1)
Hence it is proved.

Question 6.
Find the 12^th term from the last term of the A.P -2, -4, -6,… -100.
Answer:
The given A.P is -2, -4, -6, …. 100
d = -4 – (-2) = -4 + 2 = – 2
Finding the 12 term from the last term
a = -100, d = 2 (taking from the last term)
n = 12
t_n = a + (n – 1)d
t₁₂ = – 100 + 11 (2)
= -100 + 22
= -78
∴ The 12^th term of the A.P from the last term is – 78

Question 7.
Two A.P’s have the same common difference. The first term of one A.P is 2 and that of the other is 7. Show that the difference between their 10th terms is the same as the difference between their 21st terms, which is the same as the difference between any two corresponding terms.
Solution:
Let the two A.Ps be
AP₁ = a₁, a₁ + d, a₁ + 2d,…
AP₂ = a₂, a₂ + d, a₂ + 2d,…
In AP₁ we have a₁ = 2
In AP₂ we have a₂ = 7
t₁₀ in AP₁ = a₁ + 9d = 2 + 9d ………….. (1)
t₁₀ in AP₂ = a₂ + 9d = 7 + 9d …………… (2)
The difference between their 10th terms
= (1) – (2) = 2 + 9d – 7 – 9d
= -5 ………….. (I)
t₂₁ m AP₁ = a₁ + 20d = 2 + 20d …………. (3)
t₂₁ in AP₂ = a₂ + 20d = 7 + 20d ………… (4)
The difference between their 21 st terms is
(3) – (4)
= 2 + 20d – 7 – 20d
= -5 ……………. (II)
I = II
Hence it is Proved.

Question 8.
A man saved ₹16500 in ten years. In each year after the first he saved ₹100 more than he did in the preceding year. How much did he save in the first year?
Answer:
Amount of saving in ten years = ₹ 16500
S₁₀ = 16500, d= 100
S_n = \(\frac { n }{ 2 } \) [2a + (n – 1)d]
S₁₀ = \(\frac { 10 }{ 2 } \) [2a + 9d]
16500 = \(\frac { 10 }{ 2 } \) [2a + 900] = 5(2a + 900)
16500 = 10a + 4500 ⇒ 16500 – 4500 = 10a
12000 = 10a
a = \(\frac { 12000 }{ 10 } \) = 1200
Amount saved in the first year = ₹ 1200

Question 9.
Find the G.P. in which the 2nd term is \(\sqrt { 6 }\) and the 6th term is 9 \(\sqrt { 6 }\).
Answer:
2^nd term of the G.P = \(\sqrt { 6 }\)
t₂ = \(\sqrt { 6 }\)
[t_n = a r^n-1]
a.r = \(\sqrt { 6 }\) ….(1)
6^th term of the G.P. = 9 \(\sqrt { 6 }\)
a. r⁵ = 9\(\sqrt { 6 }\) ……..(2)

Question 10.
The value of a motorcycle depreciates at a rate of 15% per year. What will be the value of the motorcycle 3 year hence, which is now purchased for ₹45,000?
Solution:
a = ₹45000
Depreciation = 15% for ₹45000
= 45000 × \(\frac { 15 }{ 100 } \)
d = ₹6750 since it is depreciation
d = -6750
At the end of 1^st year its value = ₹45000 – ₹6750
= ₹38250,
Again depreciation = 38250 × \(\frac { 15 }{ 100 } \) = 5737.50
At the end of 2^nd year its value
= ₹38250 – ₹5737.50 = 32512.50
Again depreciation = 32512.50 × \(\frac { 15 }{ 100 } \) = 4876.88
At the end of the 3^rd year its value
= 32512.50 – 4876.88 = 27635.63
∴ The value of the automobile at the 3^rd year
= ₹ 27636

Must Read:

UBL Pivot Point Calculator

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 2 Integral Calculus I Ex 2.6 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 2 Integral Calculus I Ex 2.6

Question 1.
Integrate the following with respect to x.
\(\frac { 2x+5 }{x^2+5x-7}\)
Solution:
∫\(\frac { 2x+5 }{x^2+5x-7}\) dx
∫\(\frac { 1 }{z}\) dz
= log |z| + c
= log |x² + 5x – 7| + c
Take z = x² + 5x – 7
\(\frac { dz }{dx}\) = 2x + 5
dz = (2x + 5)dx

Question 2.
\(\frac { e^{3logx} }{x^4+1}\)
Solution:

Question 3.
\(\frac { e^{2x} }{e^{2x}-2}\)
Solution:

Question 4.
\(\frac { (logx)^3 }{x}\)
Solution:

Question 5.
\(\frac { 6x+7 }{\sqrt{3x^2+7x-1}}\)
Solution:

Question 6.
(4x + 2) \(\sqrt {x^2+x+1}\)
Solution:

Question 7.
x⁸ (1 + x⁹)⁵
Solution:

Question 8.
\(\frac { x^{e-1}+e^{x-1} }{x^e+e^x}\)
Solution:

Question 9.
\(\frac { 1 }{x log x}\)
Solution:
∫\(\frac { 1 }{x log x}\) dx
∫\(\frac { 1 }{z}\) dz
= log |z| + c
= log |log x| + c
Take z = log x
\(\frac { 1 }{z}\) = \(\frac { 1 }{x}\)
dz = \(\frac { 1 }{x}\) dx

Question 10.
\(\frac { x }{2x^4-3x^2-2}\)
Solution:

Question 11.
e^x (1 + x) log (xe^x)
Solution:
e^x (1 + x) log(x e^x) = (e^x + x e^x) log (x e^x)
Let z = x e^x, Then dz = d(x e^x)
dz = (x e^x + e^x) dx (Using product rule)
So ∫ e^x (1 + x) log (x e^x) dx
= ∫ log (x e^x) (e^x + x e^x) dx
= ∫ log z dz
= z (log z – 1) + c
= x e^x [log (x e^x) – 1] + c

Question 12.
\(\frac { 1 }{x(x^2+1)}\)
Solution:

Put x = 0
1 = A(1)
A = 1
Put x = 1
1 = A(2) + 1(B + C)
1 = (1)2 + B + C
B + C = -1 …….. (1)
Put x = -1
1 = A[(-1)² + 1] + (-1)[B(-1) + C]
1 = A(2) – (-B + C)
1 = 2A + B – C
1 = 2(1) + B – C
B – C = -1 ………. (2)
Adding (1) & (2)
2B = -2
B = -1
C = 0

Question 13.
e^x [ \(\frac { 1 }{x^2}\) – \(\frac { 2 }{x^3}\) ]
Solution:
∫e^x [ \(\frac { 1 }{x^2}\) – \(\frac { 2 }{x^3}\) ] dx
= ∫e^x [f(x) + f'(x)] dx
= e^x f(x) + c
= e^x [ \(\frac { 1 }{x^2}\) ] + c
Take
f(x) = \(\frac { 1 }{x^2}\)
f'(x) = \(\frac { -2 }{x^3}\)

Question 14.
e^x [ \(\frac { x-1 }{(x+1)^3}\) ]
Solution:

Question 15.
e^3x [ \(\frac { 3x-1 }{9x^2}\) ]
Solution:

Read More:

MARUTI Pivot Point Calculator

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 2 Integral Calculus I Ex 2.7 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 2 Integral Calculus I Ex 2.7

Question 1.
Integrate the following with respect to x.
\(\frac { 1 }{9-16x^2}\)
Solution:

Question 2.
\(\frac { 1} {9-8x-x^2}\)
Solution:

By Completing the squares
9 – 8x – x²
= -(x² + 8x – 9)
= – [x² + 8x + (4)² – (4)² – 9]
= – [(x + 4)² – 25]
= [25 – (x + 4)²]
= (5)² – (x + 4)²

Question 3.
\(\frac { 1 }{2x^2-9}\)
Solution:

Question 4.
\(\frac { 1 }{x^2-x-2}\)
Solution:

Question 5.
\(\frac { 1 }{x^2+3x+2}\)
Solution:

Question 6.
\(\frac { 1 }{2x^2+6x-8}\)
Solution:

Question 7.
\(\frac { e^x }{e^2x-9}\)
Solution:

Question 8.
\(\frac { 1 }{\sqrt {9x^2-7}} \)
Solution:

Question 9.
\(\frac { 1 }{\sqrt {x^2+16x+13}} \)
Solution:
∫\(\frac { 1 }{\sqrt {x^2+16x+13}} \) dx
= ∫\(\frac { 1 }{\sqrt {(x+3)^2+(2)^2}} \) dx
= log |(x + 3) + \(\sqrt {(x+3)^2+(2)^2}\)| + c
= log |(x + 3) + \(\sqrt {x^2+16x+13}\)| + c
By Completing the squares
x² + 6x + 3 = x² + 6x + (3)² – (3)² + 13
= (x + 3)² – 9 + 13
= (x + 3)² + 4
= (x + 3)² + (2)²

Question 10.
\(\frac { 1 }{ \sqrt x^2-3x+2 }\)
Solution:

Question 11.
\(\frac { x^3 }{ \sqrt x^8-1 }\)
Solution:

Question 12.
\(\sqrt { 1 + x + x^2}\)
Solution:

Question 13.
\(\sqrt { x^2 -2}\)
Solution:

Question 14.
\(\sqrt { 4x^2 -5}\)
Solution:

Question 15.
\(\sqrt { 2x^2 +4x+1}\)
Solution:

Question 16.
\(\frac { 1 }{ x + \sqrt x^2-1 }\)
Solution:

Must Read:

L&TFH Pivot Point Calculator

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 2 Integral Calculus I Ex 2.8 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 2 Integral Calculus I Ex 2.8

Using second fundamental theorem, evaluate the following:

Question 1.
\(\int_{0}^{1}\) e^2x dx
Solution:

Question 2.
\(\int_{0}^{1/4}\) \(\sqrt { 1 -4x}\) dx
Solution:

Question 3.
\(\int_{0}^{1}\) \(\frac { xdx }{x^2+1}\)
Solution:

Question 4.
\(\int_{0}^{3}\) \(\frac { e^xdx }{1+e^x}\)
Solution:
\(\int_{0}^{3}\) \(\frac { e^xdx }{1+e^x}\)
= {log |1 + ex|}\(_{0}^{3}\)
= log |1 + e³| – log |1 + e°|
= log |1 + e³| – log |1 + 1|
= log |1 + e³| – log |2|
= log |\(\frac { 1+e^3 }{2}\)|

Question 5.
\(\int_{0}^{1}\) xe^x² dx
Solution:

Question 6.
\(\int_{1}^{e}\) \(\frac { dx }{x(1+logx)^3}\)
Solution:

Question 7.
\(\int_{-1}^{1}\) \(\frac { 2x+3 }{x^2+3x+7}\) dx
Solution:

Question 8.
\(\int_{0}^{π/2}\) \(\sqrt { 1 +cosx} \) dx
Solution:

Question 9.
\(\int_{1}^{2}\) \(\frac { x-1 }{x^2}\) dx
Solution:

Evaluate the following

Question 10.
\(\int_{1}^{4}\) f(x) dx where f(x) = \(\left\{\begin{array}{l}
4 x+3,1 \leq x \leq 2 \\
3 x+5,2 \end{array}\right.\)
Solution:
\(\int_{1}^{4}\) f(x) dx
= \(\int_{1}^{2}\) f(x) dx + \(\int_{2}^{4}\) f(x) dx
= \(\int_{1}^{2}\) (4x + 3) dx + \(\int_{2}^{4}\) (3x + 5) dx

(8 + 6) – [5] + [24 + 20] – [6 + 10]
= 14 – 5 + 44 – 16
= 58 – 21
= 37

Question 11.
\(\int_{0}^{2}\) f(x) dx where f(x) = \(\left\{\begin{array}{ll}
3-2 x-x^{2}, & x \leq 1 \\
x^{2}+2 x-3, & 1<x \leq 2
\end{array}\right.\)
Solution:
\(\int_{0}^{2}\) f(x) dx
= \(\int_{0}^{1}\) f(x) dx + \(\int_{1}^{2}\) f(x) dx
= \(\int_{0}^{1}\) (3 – 2x – x²) dx + \(\int_{1}^{2}\) (x² + 2x – 3) dx

Question 12.
\(\int_{-1}^{1}\) f(x) dx where f(x) = \(\left\{\begin{array}{ll}
x, & x \geq 0 \\
-x, & x<0
\end{array}\right.\)
Solution:
\(\int_{-1}^{1}\) f(x) dx
\(\int_{-1}^{0}\) f(x) dx + \(\int_{0}^{1}\) f(x) dx
= \(\int_{-1}^{0}\) (-x) dx + \(\int_{0}^{1}\) x dx

Question 13.
f(x) = \(\left\{\begin{array}{l}
c x, \quad 0<x<1 \\
0, \text { otherwise }
\end{array}\right.\) find ‘c’ if \(\int_{0}^{1}\) f(x) dx = 2
Solution:
Given
f(x) = \(\left\{\begin{array}{l}
c x, \quad 0<x<1 \\
0, \text { otherwise }
\end{array}\right.\)
⇒ \(\int_{0}^{1}\) f(x) dx = 2
⇒ \(\int_{0}^{1}\) cx dx = 2
c[ \(\frac { x^2 }{ 2 }\) ]\(_{0}^{1}\) = 2
c[ \(\frac { 1 }{ 2 }\) – 0 ] = 2
\(\frac { 1 }{ 2 }\) = 2
⇒ c = 4

Read More:

CUMMINSIND Pivot Point Calculator

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 2 Integral Calculus I Ex 2.9 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 2 Integral Calculus I Ex 2.9

Evaluate the following using properties of definite integrals:

Question 1.
\(\int_{-π/4}^{π/4}\) x³ cos³ x dx
Solution:
Let f(x) = x³cos³x
f(-x) = (-x)³ cos³(-x)
= -x³ cos³x
f(-x) = -f(x)
⇒ f(x) is an odd function
∴ \(\int_{-π/4}^{π/4}\) x³ cos³ x dx = 0

Question 2.
\(\int_{-π/2}^{π/2}\) sin² θ dθ
Solution:
Let f(θ)= sin² θ
f(-θ) = sin² (-θ) = [sin (-θ)]²
= [-sin θ]² = sin² θ
f(-θ) = f(θ)
∴ f(θ) is an even function

Question 3.
\(\int_{-1}^{1}\) log(\(\frac { 2-x }{2+x}\)) dx
Solution:

Question 4.
\(\int_{0}^{π/2}\) \(\frac { sin^7x }{sin^7x+cos^7x}\) dx
Solution:
Using the property
\(\int_{0}^{a}\) f(x) dx = \(\int_{0}^{a}\) f(a – x) dx

Question 5.
\(\int_{0}^{1}\) log (\(\frac { 1 }{x}\) – 1) dx
Solution:

Question 6.
\(\int_{0}^{1}\) \(\frac { x }{(1-x)^{3/4}}\) dx
Solution:
Let I = \(\int_{0}^{1}\) log \(\frac { x }{(1-x)^{3/4}}\) dx
Using the property
\(\int_{0}^{a}\) f(x) dx = \(\int_{0}^{a}\) f(a – x) dx

Read More:

BOSCHLTD Pivot Point Calculator

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 3 Integral Calculus II Ex 3.2 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 3 Integral Calculus II Ex 3.2

Question 1.
The cost of an overhaul of an engine is Rs 10,000 The Operating cost per hour is at the rate of 2x-240 where the engine has run x km. find out the total cost of the engine run for 300 hours after overhaul.
Solution:
Given that the overhaul cost is Rs. 10,000.
The marginal cost is 2x – 240
MC = 2x – 240
C = ∫ MC dx + k
C = x² – 240x + k
k is the overhaul cost
⇒ k = 10,000
So C = x² – 240x + 10,000
When x = 300 hours, total cost is
C = (300)² – 240(300) + 10,000
⇒ C = 90,000 – 72000 + 10,000
⇒ C = 28,000
So the total cost of the engine run for 300 hours after the overhaul is ₹ 28,000.

Question 2.
Elasticity of a function \(\frac { Ey }{Ex}\) is given by \(\frac { Ey }{Ex}\) = \(\frac { -7x }{(1-2x)(2+3x)}\). Find the function when x = 2, y = \(\frac { 3 }{8}\)
Solution:

Put x = 0
7 = A (3(0) + 2) + B (2(0) – 1)
7 = A (2) + B (-1)
7 = (2) (2) – B
B = 4 – 7
B = -3

Question 3.
The Elasticity of demand with respect to price for a commodity is given by where \(\frac { (4-x)}{x}\) p is the price when demand is x. find the demand function when the price is 4 and the demand is 2. Also, find the revenue function
Solution:
The elasticity at the demand

Integrating on both sides
∫\(\frac { 1}{(x-4)}\) = ∫\(\frac { 1}{p}\) dp
log |x – 4| = log |p| + log k
log |x – 4| = log |pk| ⇒ (x – 4) = pk ……… (1)
when p = 4 and x = 2
(2 – 4) = 4k ⇒ -2 = 4k
k = -1/2
Eqn (1) ⇒ (x – 4) = p(-1/2)
-2 (x – 4) = p ⇒ p = 8 – 2x
Revenue function R = px = (8 – 2x)x
R = 8x – 2x²

Question 4.
A company receives a shipment of 500 scooters every 30 days. From experience it is known that the inventory on hand is related to the number of days x. Since the shipment, I (x) = 500 – 0.03 x², the daily holding cost per scooter is Rs 0.3. Determine the total cost for maintaining inventory for 30 days
Solution:
Here I (x) = 500 – 0.03 x²
C¹ = Rs 0.3
T = 30
Total inventory carrying cost
= C¹ \(\int _{0}^{T}\) I(x) dx
= 0.3 \(\int _{0}^{30}\) (500 – 0.03 x²)dx
= 0.3 [500 x – 0.03(\(\frac { x^3 }{3}\))]\( _{0}^{30}\)
= 0.3 [ 500 x – 0.01 x³]\( _{0}^{30}\)
= 0.3 [500(30) – 0.01 (30)³] – [0]
= 0.3 [15000 – 0.01 (27000)]
= 0.3 [15000 – 270] = 0.3 [14730]
= Rs 4,419

Question 5.
An account fetches interest at the rate of 5% per annum compounded continuously an individual deposits Rs 1000 each year in his account. how much will be in the account after 5 years (e^0.25 = 1.284)
Solution:
P = 1000
r = \(\frac { 5 }{1000}\) = 0.05
N = 5
Annuity = \(\int _{0}^{5}\) 1000 e^0.05t dt
= 1000 [ \(\frac { e^{0.05t} }{0.05}\) ] \(_{0}^{5}\)
= \(\frac { 1000 }{0.05}\) [e^0.05(5) – e⁰]
= 20000 [e^0.25 – 1]
= 20000 [1.284 – 1]
= 20000 [0.284]
= Rs 5680

Question 6.
The marginal cost function of a product is given by \(\frac { dc }{dx}\) = 100 – 10x + 0.1 x² where x is the output. Obtain the total and average cost function of the firm under the assumption, that its fixed cost is t 500
Solution:
\(\frac { dc }{dx}\) = 100 – 10x + 0.1 x² and k = Rs 500
dc = (100 – 10x + 0.1 x²) dx
Integrating on both sides,

Question 7.
The marginal cost function is M.C = 300 x^2/5 and the fixed cost is zero. Find the total cost as a function of x
Solution:
M.C = 300 x^2/5 and fixed cost K = 0
Total cos t = ∫M.C dx

Question 8.
If the marginal cost function of x units of output is \(\frac { a }{\sqrt {ax+b}}\) and if the cost of output is zero. Find the total cost as a function of x.
Solution:

∴ C(x) = 2(ax + b)^1/2 + k …….. (1)
When x = 0
eqn (1) ⇒ 0 = 2 [a(0) + b]^1/2 + k
k = -2(b)^1/2 ⇒ k = -2√b
Required cost function
C(x) = 2(ax + b)^1/2 – 2√b
∴ C = 2\(\sqrt { ax + b}\) – 2√b

Question 9.
Determine the cost of producing 200 air conditioners if the marginal cost (is per unit) is C'(x) = \(\frac { x^2 }{200}\) + 4
Solution:

= 13333.33 + 800
∴ Cost of producing 200 air conditioners
= Rs 14133.33

Question 10.
The marginal revenue (in thousands of Rupees) function for a particular commodity is 5 + 3 e^-0.03x where x denotes the number of units sold. Determine the total revenue from the sale of 100 units (given e^-3 = approximately)
Solution:
The marginal Revenue (in thousands of Rupees) function
M.R = 5 + 3^-0.03x
Total Revenue from sale of 100 units is
Total Revenue T.R = \(\int _{0}^{ 100}\) M.R dx

= [500 – 100 e^-3] – [0 – 100 e°]
= [500 -100 (0.05)] – [-100 (1)]
= [500 – 5]+ 100
= 495 + 100 = 595 thousands
= 595 × 1000
∴ Revenue R = Rs 595000

Question 11.
If the marginal revenue function for a commodity is MR = 9 – 4x². Find the demand function.
Solution:
Marginal Revenue function MR = 9 – 4x²
Revenue function R = ∫MR dx

Question 12.
Given marginal revenue function \(\frac { 4 }{(2x+3)^2}\) -1, show that the average revenue function is P = \(\frac { 4 }{6x+9}\) -1
Solution:
M.R = \(\frac { 4 }{(2x+3)^2}\) -1
Total Revenue R = ∫M.R dx

Question 13.
A firms marginal revenue functions is M.R = 20 e^-x/10 Find the corresponding demand function.
Solution:

Question 14.
The marginal cost of production of a firm is given by C’ (x) = 5 + 0.13 x, the marginal revenue is given by R’ (x) = 18 and the fixed cost is Rs 120. Find the profit function.
Solution:
MC = C'(x) = 5 + 0.13x
C(x) = ∫C'(x) dx + k₁
= ∫(5 + 0.13x) dx + k₁
= 5x + \(\frac{0.13}{2} x^{2}\) + k₁
When quantity produced is zero, fixed cost is 120
(i.e) When x = 0, C = 120 ⇒ k₁ = 120
Cost function is 5x + 0.065x² + 120
Now given MR = R'(x) = 18
R(x) = ∫18 dx + k₂ = 18x + k₂
When x = 0, R = 0 ⇒ k₂ = 0
Revenue = 18x
Profit P = Total Revenue – Total cost = 18x – (5x + 0.065x² + 120)
Profit function = 13x – 0.065x² – 120

Question 15.
If the marginal revenue function is R'(x) = 1500 – 4x – 3x². Find the revenue function and average revenue function.
Solution:
Given marginal revenue function
MR = R’(x)= 1500 – 4x – 3x²
Revenue function R(x) = ∫R'(x) dx + c
R = ∫(1500 – 4x – 3x²) dx + c
R = 1500x – 2x² – x³ + c
When x = 0, R = 0 ⇒ c = 0
So R = 1500x – 2x² – x³
Average revenue function P = \(\frac{R}{x}\) ⇒ 1500 – 2x – x²

Question 16.
Find the revenue function and the demand function if the marginal revenue for x units MR = 10 + 3x – x
Solution:
The marginal revenue function
MR = 10 + 3x – x²
The Revenue function
R = ∫(MR) dx
= ∫(10 + 3x – x²)dx

Question 17.
The marginal cost function of a commodity is given by M_c = \(\frac { 14000 }{\sqrt{7x+4}}\) and the fixed cost is Rs 18,000. Find the total cost average cost.
Solution:
The marginal cost function of a commodity
M_c = \(\frac { 14000 }{\sqrt{7x+4}}\) = 14000 (7x + 4)^-1/2
Fixed cost k = Rs 18,000
Total cost function C = ∫(M.C) dx
= ∫14000 (7x + 4)^-1/2 dx

Question 18.
If the marginal cost (MC) of production of the company is directly proportional to the number of units (x) produced, then find the total cost function, when the fixed cost is Rs 5,000 and the cost of producing 50 units is Rs 5,625.
Solution:
M.C αx
M.C = λx
fixed cost k = Rs 5000
Cost function C = ∫(M.C) dx
= ∫λx dx

Question 19.
If MR = 20 – 5x + 3x², Find total revenue function
Solution:
MR = 20 – 5x + 3x²
Total Revenue function
R = ∫(MR) dx = ∫(20 – 5x + 3x²) dx
R = 20x – \(\frac { 5x^2 }{2}\) + \(\frac {3x^3 }{3}\) + k
when x = 0; R = 0 ⇒ k = 0
∴ R = 20x – \(\frac { 5 }{2}\) x² + x³

Question 20.
If MR = 14 – 6x + 9x², Find the demand function.
Solution:
MR = 14 – 6x + 9x²
R = ∫(14 – 6x + 9x²) dx + k
= 14x – 3x² + 3x³ + k
Since R = 0, when x = 0, k = 0
So revenue function R = 14x – 3x² + 3x³
Demand function P = \(\frac{R}{x}\) = 14 – 3x + 3x²

Read More:

• ABFRL Pivot Point Calculator

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 3 Integral Calculus II Ex 3.1 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 3 Integral Calculus II Ex 3.1

Question 1.
Using Interation, find the area of the region bounded the line given is 2y + x = 8, the x axis and the lines x = 2, x = 4.
Solution:
The equation of the line given is 2y + x = 8
⇒ 2y = 8 – x ⇒ y = \(\frac { 8-x }{2}\)
y = 4 – \(\frac { x }{2}\)
Also x varies from 2 to 4
The required Area

= (16 – 4) – (8 – 1)
= 12 – 7 = 5 sq.units

Question 2.
Find the area bounded by the lines y – 2x – 4 = 0, y = 0, y = 3 and the y-axis.
Solution:
The equation of the line given is y – 2x – 4 = 0
⇒ 2x = y – 4 ⇒ x = \(\frac { y-4 }{2}\)
∴ x = \(\frac { y }{2}\) – 2
Also y varies from 1 to 3
Required Area

= 2 – 4 = -2
Area can’t be in negative.
∴ Area = 2 sq.units

Question 3.
Calculate the area bounded by the parabola y² = 4ax and its latus rectum.
Solution:
Given parabola is y² = 4ax
Its focus is (a, 0)
Equation of latus rectum is x = a
The parabola is symmetrical about the x-axis

Question 4.
Find the area bounded by the line y = x and x-axis and the ordinates x = 1, x = 2
Solution:
The equation of the given line is y = x and x varies from 1 to 2

Question 5.
Using integration, find the area of the region bounded by the line y – 1 = x, the x-axis and the ordinates x = -2, x = 3.
Solution :
The equation of given line is y – 1 = x
y = x + 1

The line y = x + 1 meets the x-axis at x = -1
Since x varies from -2 to 3
Hence a part of lies below the x-axis and the other part lies above the x-axis.

Question 6.
Find the area of the region lying in the first quadrant bounded by the region y = 4x², x = 0, y = 0 and y = 4
Solution:
The equation of a parabola given is y = 4x²

Area of the region lying in the first quadrant

Question 7.
Find the area bounded by the curve y = x² and the line y = 4
Solution:
Equation of the curve y = x² ………. (1)
Equation of the line y = 4 ………. (2)
Solving equation (1) & (2)
x² = 4 ⇒ x = ± 2

Must Read:

Gift Nifty Timings

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 2 Integral Calculus I Ex 2.12 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 2 Integral Calculus I Ex 2.12

Choose the most suitable answer from the given four alternatives:

Question 1.
∫\(\frac { 1 }{x^3}\) dx is
(a) \(\frac { -3 }{x^2}\) + c
(b) \(\frac { -1 }{2x^2}\) + c
(c) \(\frac { -1 }{3x^2}\) + c
(d) \(\frac { -2 }{x^2}\) + c
Solution:
(b) \(\frac { -1 }{2x^2}\) + c
Hint:
∫\(\frac { 1 }{x^3}\) dx = ∫x^-3 dx = [ \(\frac { x^{-3+1} }{-3+1}\) ] + c
= (\(\frac { x^{-2} }{-2}\)) + c = \(\frac { -1 }{2x^2}\) + c

Question 2.
∫2^x dx is
(a) 2^x log 2 + c
(b) 2^x + c
(c) \(\frac { 2^x }{log 2}\) + c
(d) \(\frac { log 2 }{2^x}\) + c
Solution:
(c) \(\frac { 2^x }{log 2}\) + c
Hint:
∫2^x dx = ∫a^x dx = \(\frac { a^x }{log a}\) + c

Question 3.
∫\(\frac { sin 2x }{2 sin x}\) dx is
(a) sin x + c
(b) \(\frac { 1 }{2}\) sin x + c
(c) cos x + c
(d) \(\frac { 1 }{2}\) cos x + c
Solution:
(a) sin x + c
Hint:
∫\(\frac { sin 2x }{2 sin x}\) dx = ∫\(\frac { 2sin x cos x }{2 sin x}\) dx
= ∫cos x dx
= sin x + c

Question 4.
∫\(\frac { sin 5x-sin x }{cos 3x}\) dx is
(a) -cos 2x + c
(b) -cos 2x – c
(c) –\(\frac { 1 }{4}\) cos 2x + c
(d) -4 cos 2x + c
Solution:
(a) -cos 2x + c
Hint:

Question 5.
∫\(\frac { log x}{x}\) dx, x > 0 is
(a) \(\frac { 1 }{2}\) (log x)² + c
(b) –\(\frac { 1 }{2}\) (log x)²
(c) \(\frac { 2 }{x^2}\) + c
(d) \(\frac { 2 }{x^2}\) – c
Solution:
(a) \(\frac { 1 }{2}\) (log x)² + c
Hint:
∫\(\frac { log x}{x}\) dx, x > 0
∫ tdt = [ \(\frac { t^2 }{2}\) ] + c
= \(\frac { (log x)^2 }{2}\) + c
let t = log x
\(\frac { dt }{dx}\) = \(\frac { 1 }{x}\)
dt = \(\frac { 1 }{x}\) dx

Question 6.
∫\(\frac { e^x }{\sqrt{1+e^x}}\) dx is
(a) \(\frac { e^x }{\sqrt{1+e^x}}\) + c
(b) 2\(\sqrt{1+e^x}\) + c
(c) \(\sqrt{1+e^x}\) + c
(d) e^x\(\sqrt{1+e^x}\) + c
Solution:
(b) 2\(\sqrt{1+e^x}\) + c
Hint:

Question 7.
∫\(\sqrt { e^x}\) dx is
(a) \(\sqrt { e^x}\) + c
(b) 2\(\sqrt { e^x}\) + c
(c) \(\frac { 1 }{2}\) \(\sqrt { e^x}\) + c
(d) \(\frac { 1 }{2\sqrt { e^x}}\) + c
Solution:
(b) 2\(\sqrt { e^x}\) + c
Hint:
∫\(\sqrt { e^x}\) dx
= ∫\(\sqrt { e^x}\) dx = ∫(e^x)^1/2 dx = ∫ e^x/2 dx
= \(\frac { e^{x/2} }{1/2}\) + c = 2e^x/2 + c
= 2(e^x)^1/2 + c = 2\(\sqrt { e^x}\) + c

Question 8.
∫e^2x [2x² + 2x] dx
(a) e^2x x² + c
(b) xe^2x + c
(c) 2x²e² + c
(d) \(\frac { x^2e^x }{2}\) + c
Solution:
(a) e^2x x² + c
Hint:
∫e^2x (2x² + 2x) dx
Let f(x) = x²; f'(x) = 2x and a = 2
= ∫e^ax [af(x),+ f ’(x)] = e^ax f(x) + c
= ∫e^2x (2x² + 2x) dx = e^2x (x²) + c

Question 9.
\(\frac { e^x }{e^x+1}\) dx is
(a) log |\(\frac { e^x }{e^x+1}\)| + c
(b) log |\(\frac { e^x+1 }{e^x}\)| + c
(c) log |e^x| + c
(d) log |e^x + 1| + c
Solution:
(d) log |e^x + 1| + c
Hint:
∫\(\frac { e^x }{e^x+1}\) dx
= ∫\(\frac { dt }{t}\)
= log |t| + c
= log |e^x + 1| + c
take t = e^x + 1
\(\frac { dt }{dx}\) = e^x
dt = e^x dx

Question 10.
∫\(\frac { 9 }{x-3}-\frac { 1 }{x+1}\) dx is
(a) log |x – 3| – log|x + 1| + c
(b) log|x – 3| + log|x + 1| + c
(c) 9 log |x – 3| – log |x + 1| + c
(d) 9 log |x – 3| + log |x + 1| + c
Solution:
(c) 9 log |x – 3| – log |x + 1| + c
Hint:

Question 11.
∫\(\frac { 2x^3 }{4+x^4}\) dx is
(a) log |4 + x⁴| + c
(b) \(\frac { 1 }{2}\) log |4 + x⁴| + c
(c) \(\frac { 1 }{2}\) log |4 + x⁴| + c
(d) log |\(\frac { 2x^3 }{4+x^4}\) + c
Solution:
(b) \(\frac { 1 }{2}\) log |4 + x⁴| + c
Hint:

Question 12.
∫\(\frac { dx }{\sqrt{x^2-36}}\) is
(a) \(\sqrt{x^2-36}\) + c
(b) log |x + \(\sqrt{x^2-36}\)| + c
(c) log |x – \(\sqrt{x^2-36}\)| + c
(d) log |x² + \(\sqrt{x^2-36}\)| + c
Solution:
(b) log |x + \(\sqrt{x^2-36}\)| + c
Hint:

Question 13.
∫\(\frac { 2x+3 }{\sqrt{x^2+3x+2}}\) dx is
(a) \(\sqrt{x^2+3x+2}\) + c
(b) 2\(\sqrt{x^2+3x+2}\) + c
(c) \(\sqrt{x^2+3x+2}\) + c
(d) \(\frac { 2 }{3}\) (x² + 3x + 2) + c
Solution:
(b) 2\(\sqrt{x^2+3x+2}\) + c
Hint:

Question 14.
\(\int_{0}^{4}\) (2x + 1) dx is
(a) 1
(b) 2
(c) 3
(d) 4
Solution:
(b) 2
Hint:
\(\int_{0}^{4}\) (2x + 1) dx
= [2(\(\frac { x^2 }{2}\)) + x]\(_{0}^{1}\) = [x² + x]\(_{0}^{1}\)
= [(1)² + (1)] – [0] = 2

Question 15.
\(\int_{2}^{4}\) \(\frac { dx }{x}\) is
(a) log 4
(b) 0
(c) log 2
(d) log 8
Solution:
(c) log 2
Hint:
\(\int_{2}^{4}\) \(\frac { dx }{x}\)
\(\int_{2}^{4}\) \(\frac { dx }{x}\) = [log |x|]\(_{0}^{1}\) = log |4| – log |2|
= log[ \(\frac { 4}{2}\) ] = log 2

Question 16.
\(\int_{0}^{∞}\) e^-2x dx is
(a) 0
(b) 1
(c) 2
(d) \(\frac { 1 }{2}\)
Solution:
(d) \(\frac { 1 }{2}\)
Hint:

Question 17.
\(\int_{-1}^{1}\) x³ e^x4 dx is
(a) 1
(b) 2\(\int_{0}^{1}\) x³ e^x4
(c) 0
(d) e^x4
Solution:
(c) 0
Hint:
\(\int_{-1}^{1}\) x³ e^x4 dx
Let f (x) = x³e^x4
f(-x) = (-x)² e^(-x)4
= -x² e^x4
f(-x) = -f(x)
⇒ f(x) is an odd function
∴ \(\int_{-1}^{1}\) x³ e^x4 dx = 0

Question 18.
If f(x) is a continuous function and a < c < b, then \(\int_{a}^{c}\) f(x) dx + \(\int_{c}^{b}\) f(x) dx is
(a) \(\int_{a}^{b}\) f(x) dx – \(\int_{a}^{c}\) f(x) dx
(b) \(\int_{a}^{c}\) f(x) dx – \(\int_{a}^{b}\) f(x) dx
(c) \(\int_{a}^{b}\) f(x) dx
(d) 0
Solution:
(c) \(\int_{a}^{b}\) f(x) dx

Question 19.
The value of \(\int_{-π/2}^{π/2}\) cos x dx is
(a) 0
(b) 2
(c) 1
(d) 4
Solution:
(b) 2
Hint:
\(\int_{-π/2}^{π/2}\) cos x dx
Let f(x) = cos x
f(-x) = cos (-x) = cos (x) = f(x)
∴ f(x) is an even function
\(\int_{-π/2}^{π/2}\) cos x dx = 2 × \(\int_{0}^{π/2}\) cos x dx
= 2 × [sin x]\(_{0}^{-π/2}\) = 2 [sin π/2 – sin 0]
= 2 [1 – 0] = 2

Question 20.
\(\int_{-π/2}^{π/2}\) \(\sqrt {x^4(1-x)^2}\) dx
(a) \(\frac { 1 }{12}\)
(b) \(\frac { -7 }{12}\)
(c) \(\frac { 7 }{12}\)
(d) \(\frac { -1 }{12}\)
Solution:
(a) \(\frac { 1 }{12}\)
Hint:

Question 21.
If \(\int_{0}^{1}\) f(x) dx = 1, \(\int_{0}^{1}\) x f(x) dx = a and \(\int_{0}^{1}\) x² f(x) dx = a², then \(\int_{0}^{1}\) (a – x)² f(x) dx is
(a) 4a²
(b) 0
(c) a²
(d) 1
Solution:
(b) 0
Hint:
\(\int_{0}^{1}\) (a – x)² f(x) dx
= \(\int_{0}^{1}\) [a² +x² – 2ax] f(x) dx
= \(\int_{0}^{1}\) a² + f (x) dx + \(\int_{0}^{1}\) x² f (x) dx – 2a\(\int_{0}^{1}\) x f(x) dx
= a²(1) + a² – 2a(a) – 2a² – 2a² = 0

Question 22.
The value of \(\int_{2}^{3}\) f(5 – x) dx – \(\int_{2}^{3}\) f(x) dx is
(a) 1
(b) 0
(c) -1
(d) 5
Solution:
(b) 0
Hint:
\(\int_{2}^{3}\) f(5 – x) dx – \(\int_{2}^{3}\) f(x) dx
Using the property
= \(\int_{2}^{3}\) f(x) dx = \(\int_{a}^{b}\) f(a + b – x) dx
= \(\int_{2}^{3}\) f (5 – x) – \(\int_{2}^{3}\) f (5 – x) dx
= 0

Question 23.
\(\int_{0}^{4}\) (√x + \(\frac { 1 }{√x}\)), dx is
(a) \(\frac { 20 }{3}\)
(b) \(\frac { 21 }{3}\)
(c) \(\frac { 28 }{3}\)
(d) \(\frac { 1 }{3}\)
Solution:
(c) \(\frac { 28 }{3}\)
Hint:

Question 24.
\(\int_{0}^{π/3}\) tan x dx is
(a) log 2
(b) 0
(c) log √2
(d) 2 log 2
Solution:
(a) log 2
Hint:
\(\int_{0}^{π/3}\) tan x dx
= ∫tan x dx
= ∫\(\frac { sin x }{cos x}\) dx
= -∫\(\frac { -sin x }{cos x}\) dx
= -log |cos x| + c
= log sec x + c
= [log (sec x)]\(_{0}^{π/3}\)
= log [(sec π/3) – log (sec 0)]
= log (2) – log (1)
= log 2 – (0) = log 2

Question 25.
Using the factorial representation of the gamma function, which of the following is the solution for the gamma function Γ(n) when n = 8
(a) 5040
(b) 5400
(c) 4500
(d) 5540
Solution:
(a) 5040
Hint:
\(\Upsilon\) (8) = 7! = 7 × 6 × 5 × 4 × 3 × 2 × 1 = 5040

Question 26.
Γ(n) is
(a) (n – 1)!
(b) n!
(c) n Γ (n)
(d) (n – 1) Γ(n)
Solution:
(a) (n – 1)!
Hint:
Γ(n) = Γ(n – 1) + 1 = (n – 1)!

Question 27.
Γ(1) is
(a) 0
(b) 1
(c) n
(d) n!
Solution:
(b) 1
Hint:
\(\Upsilon\) (1) = 0! = 1

Question 28.
If n > 0, then Γ(n) is
(a) \(\int_{0}^{1}\) e^-x x^n-1 dx
(b) \(\int_{0}^{1}\) e^-x xⁿ dx
(c) \(\int_{0}^{∞}\) e^x x^-n dx
(d) \(\int_{0}^{∞}\) e^-x x^n-1 dx
Solution:
(d) \(\int_{0}^{∞}\) e^-x x^n-1 dx

Question 29.
Γ(\(\frac { 3 }{2}\))
(a) √π
(b) \(\frac { √π }{2}\)
(c) 2√π
(d) \(\frac { 3 }{2}\)
Solution:
(b) \(\frac { √π }{2}\)
Hint:
\(\Upsilon\) (3/2) = \(\frac { 2 }{2}\) \(\Upsilon\) [ \(\frac { 3 }{2}\) ]
= \(\frac { 3 }{2}\) √π

Question 30.
\(\int_{0}^{∞}\) x⁴ e^-x dx is
(a) 12
(b) 4
(c) 4!
(d) 64
Solution:
(b) \(\frac { √π }{2}\)
Hint:
\(\int_{0}^{∞}\) x⁴ e^-x dx
= ∫xⁿ e^-ax dx = \(\frac { n! }{a{n+1}}\)
= \(\frac { 4! }{(1)^{n+1}}\)
= \(\frac { 4! }{(1)^5}\)
= 4!

Try More:

Moving Average Crossover Scanner

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Business Maths Guide Pdf Chapter 2 Integral Calculus I Miscellaneous Problems Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Business Maths Solutions Chapter 2 Integral Calculus I Miscellaneous Problems

Question 1.
∫\(\frac { 1 }{\sqrt{x-1}-\sqrt{x+3}}\) dx
Solution:
∫\(\frac { 1 }{\sqrt{x+2}-\sqrt{x+3}}\) dx
Conjugating the Denominator

Question 2.
∫\(\frac { dx }{2-3x-2x^2}\)
Solution:

Question 3.
∫\(\frac { dx }{e^x+6+5e^{-x}}\)
Solution:

Question 4.
∫\(\sqrt { 2x^2-3}\) dx
Solution:

Question 5.
∫\(\sqrt { 9x^2+12x+3}\) dx
Solution:

Question 6.
∫(x + 1)² log x dx
Solution:
∫udv = uv – ∫vdu
∫(x + 1)² log x dx

Question 7.
∫log (x – \(\sqrt { x^2-1}\)) dx
Solution:

Question 8.
\(\int_{0}^{1}\) \(\sqrt { x(x-1)}\) dx
Solution:

Question 9.
\(\int_{-1}^{1}\) x² e^-2x dx
Solution:

Question 10.
\(\int_{0}^{3}\) \(\frac { xdx }{\sqrt {x+1} + \sqrt{5x+1}}\)
Solution:

Must Try:

Nr7 stocks