Students can download Maths Chapter 1 Numbers Ex 1.6 Questions and Answers, Notes, Samacheer Kalvi 6th Maths Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus, helps students complete homework assignments and to score high marks in board exams.
Tamilnadu Samacheer Kalvi 6th Maths Solutions Term 1 Chapter 1 Numbers Ex 1.6
Miscellaneous Practice Problems
Question 1.
Try to open my locked suitcase which has the biggest 5 digit odd number as the password comprising the digits 7, 5, 4, 3 and 8. Find the password.
Solution:
87543
Question 2.
As per the census of 2001, the population of four states are given below. Arrange the states in ascending and descending order of their population.

Solution:
Ascending Order: 6,85,48,437 < 7,21,47,030 < 7,26,26,809 < 9,12,76,115
Descending Order 9,12,76,115 > 7,26,26,809 > 7,21,47,030 > 6,85,48,437
![]()
Question 3.
Study the following table and answer the questions.
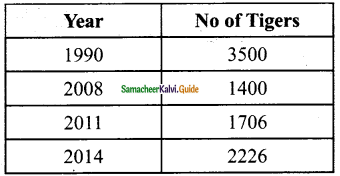
(i) How many tigers were there in 2011?
(ii) How many tigers were less in 2008 than in 1990?
(iii) Did the number of tigers increase or decrease between 2011 and 2014? If yes, by how much?
Solution:
(i) 1706
(ii) 2100
(iii) Yes, 2226 – 1706 = 520 tigers increased from 2011 to 2014
Question 4.
Mullaikodi has 25 bags of apples. In each bag, there are 9 apples. She shares them equally amongst her 6 friends. How many apples does each get? Are there any apples left over?
Solution:
No of apple bags = 25
Apples in each bag = 9
Total no of apples = 25 × 9 = 225
Apples shared among her 6 friends = 225 ÷ 6
So, among her 6 friends, each of them gets 37 apples and 3 apples are leftover.
![]()
Question 5.
Poultry has produced 15472 eggs and fits 30 eggs in a tray. How many trays do they need?
Solution:
No of eggs produced = 15472
No of eggs fits in a tray = 30
No of trays required = 15472 ÷ 30
Trays required = 515 + 1 (to fit the remaining 22 eggs) = 516
Quotient = 515
Remainder = 22
![]()
Challenging Problems
Question 6.
Read the table and answer the following questions.
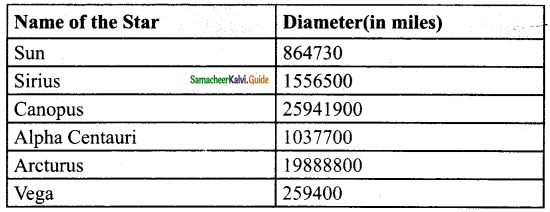
(i) Write the Canopus star’s diameter in words, in the Indian and the International System.
(ii) Write the sum of the place values of 5 in Sirius star’s diameter in the Indian System.
(iii) Eight hundred sixty four million seven hundred thirty. Write in Indian System.
(iv) Write the diameter in words of Arcturus star in the International System
(v) Write the difference of the diameters of Canopus and Arcturus stars in the Indian and the International Systems.
Solution:
(i) 2,59,41,900
25,941,900
Indian System: Two crores fifty-nine lakh forty-one thousand nine hundred.
International System: Twenty-five million nine hundred forty-one thousand nine hundred.
(ii) 5,50,500
(iii) 864,000,730 (86,40,00,730)
(iii) Eighty-six crore forty lakh seven hundred thirty.
(iv) Nineteen million eight hundred eighty-eight thousand eight hundred. (19,888,800)
(v) Indian System: 60,53,100 – Sixty lakh fifty-three thousand one hundred International System: 6,053,100 – Six million fifty-three thousand one hundred.
![]()
Question 7.
Anbu asks Arivu Selvi to guess a five-digit odd number. He gives the following hints.
The digit in the 1000s place is less than 5
The digit in the 100s place is greater than 6
The digit in the 10s place is 8.
What is Arivu Selvi’s answer? Does she give more than one answer?
Solution:
There are more than one answers.
One of them is 54781
Some of the other numbers maybe 64783, 74785, 84787 and so on.
Question 8.
A Music concert is taking place in a stadium. A total of 7,689 chairs are to be put in rows of 90.
(i) How many rows will there be?
(ii) Will there be any chairs left over?
Solution:
Total no of chairs to be put = 7,689
Chairs in each row = 90
No of rows = 7689 ÷ 90
Hence, 84 + 1 = 85 rows are required to fill 7650 chairs
Chairs left over = 79 (If the no of rows = 84)
![]()
Question 9.
Round off the seven-digit number 29,75,842 to the nearest lakhs and ten lakhs. Are they the same?
Solution:
Yes. Both are same (30,00,000)
Question 10.
Find the 5 or 6 or 7 digit numbers from a newspaper or a magazine to get a rounded number to the nearest ten thousand.
Solution:
(i) 14276 \(\simeq\) 10000
(ii) 1,86945 \(\simeq\) 1,90000
![]()