Samacheer Kalvi 12th Maths Guide Chapter 10 Ordinary Differential Equations Ex 10.7

Tamilnadu State Board New Syllabus Samacheer Kalvi 12th Maths Guide Pdf Chapter 10 Ordinary Differential Equations Ex 10.7 Textbook Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 12th Maths Solutions Chapter 10 Ordinary Differential Equations Ex 10.7

Question 1.
Solve the following Linear differential equations.
cos x \(\frac { dy }{ dx }\) + y sin x = 1
Solution:
The given differential equation can be written as

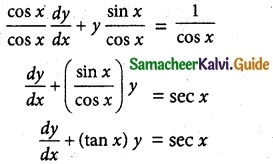
This is the form \(\frac { dy }{ dx }\) + py = Q
where P = tan x
Q = sec x
Thus, the given differential equation is linear.
I.F = e^{∫ pdx} = e^{∫ tan x dx} = e^{log (sec x)} = sec x
So, the required solution is given by
[y × I.F] = ∫ [Q × IF] dx + c
y × sec x = ∫ sec x × sec x dx + c
y sec x = ∫ sec² x dx + c
y sec x = tan x + c

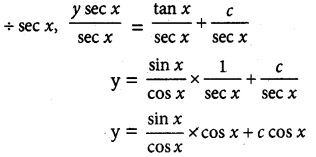
= sin x + c cos x
y = sin x + c cos x is the required solution.

Question 2.
(1 – x²)\(\frac { dy }{ dx }\) – xy = 1
Solution:
The given differential equation can be written as

Thus, the given differential equation is linear.

Which is a required solution.

Question 3.
\(\frac { dy }{ dx }\) + \(\frac { y }{ x }\) = sin x
Solution:
The given differential equation can be written as
\(\frac { dy }{ dx }\) + (\(\frac { 1 }{ x }\))y = sin x
This is of the form \(\frac { dy }{ dx }\) + Py = Q
where P = \(\frac { 1 }{ x }\)
Q = sin x
Thus, the given differential equation is linear.
I.F= e^{∫ pdx} = e^{∫ \(\frac { 1 }{ x }\) dx} = e^{log x} = x
So, the required solution is given by
yx I.F = ∫ (Q × I.F) dx + c
yx = ∫ sin x × x dx + c
= x (-cos x) – (1) (-sin x) + c
yx = -x cos x + sin x + c
yx + x cos x = sin x + c
(y + cos x) x = sin x + c is a required solution.

Question 4.
(x² + 1)\(\frac { dy }{ dx }\) + 2xy = \(\sqrt { x^2+4 }\)
Solution:
The given differential equation may be written as

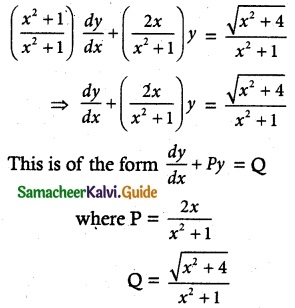
Thus, the given differential equation is linear.
I.F= e^{∫ pdx} = e^{∫ \(\frac { 2x }{ x^+1 }\) dx} = e^{log (x² + 2)} = x² + 1
So, the required solution is given by

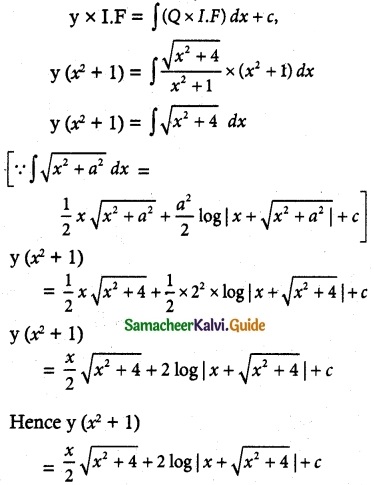
Is the required solution.

Question 5.
(2x – 10y³) dy + y dx = 0
Solution:
The given differential equation may be written as
y dx = -(2x – 10y³) dy

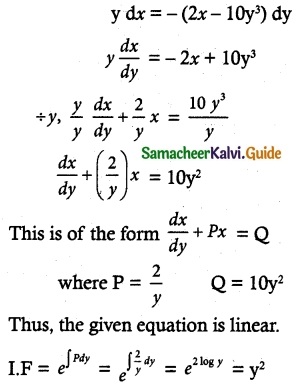
So, the required solution is
x × I.F = ∫ (Q × I.F)dy + c
xy² = ∫ 10 y² × y² dy + c
= ∫ 10 y⁴ dy + c
= \(\frac { 10y^5 }{ 5 }\) + c = 2y⁵ + c
xy² = 2y⁵ + c is a required solution.

Question 6.
x sin x \(\frac { dy }{ dx }\) + (x cos x + sin x) y = sin x
Solution:
The given differential equation can be written as

Thus, the given differential equation is linear.
I.F = e^{∫ pdx} = e^{∫ (cot x + 1/x)dx} = e^{log sin x + log x}
= e^{log (x sin x)} = x sin x
So, the solution of the given differential equation is given by
y × I.F = ∫(Q × I.F) dx + c
y (x sin x) = ∫ \(\frac { 1 }{ x }\) x sin x dx + c
= ∫ sin x dx + c
y (x sin x) = -cos x + c
xy sin x + cos x = c is the required solution.

Question 7.
Solve (y – e^sin-1x) \(\frac { dx }{ dy }\) + \(\sqrt { 1-x^2 }\) = 0
Solution:

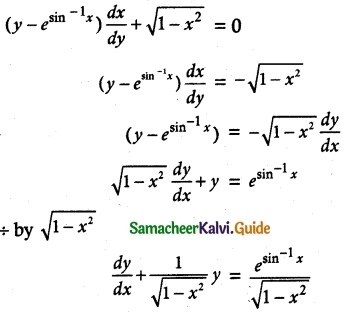
Thus, the given differential equation is Linear

Question 8.
\(\frac { dy }{ dx }\) + \(\frac { y }{ (1-x)√x }\) = 1 – √x
Solution:
The given linear differential equation is of the form
\(\frac { dy }{ dx }\) + py = Q

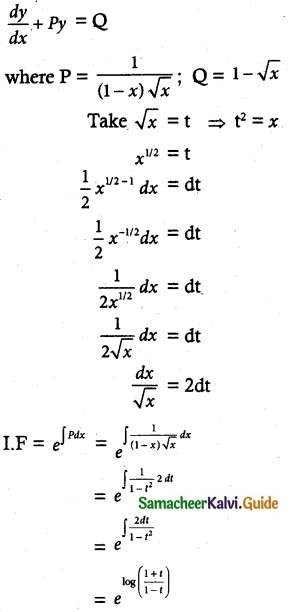
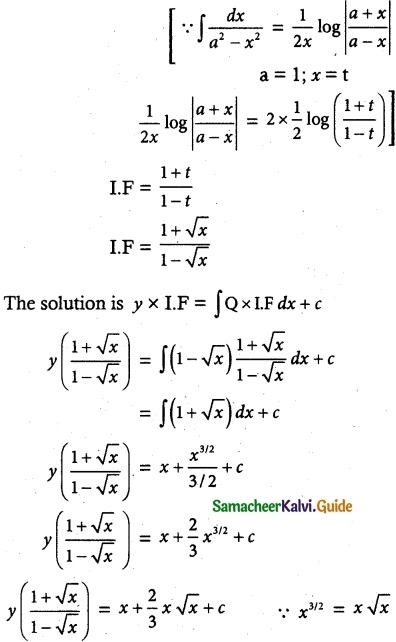
Question 9.
(1 + x + xy²) \(\frac { dy }{ dx }\) + (y + y³) = 0
Solution:

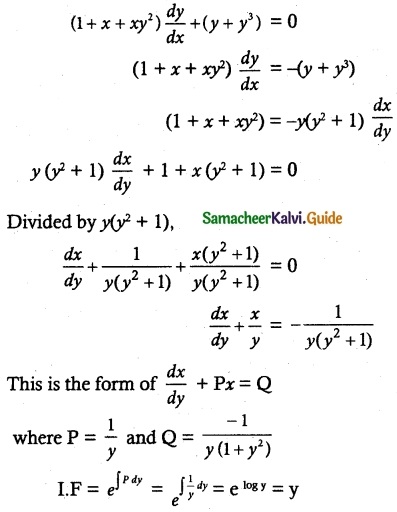
So, the solution of the equation is given by

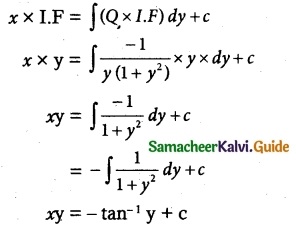
xy + tan^-1 y = c
Which is the required solution.

Question 10.
\(\frac { dy }{ dx }\) + \(\frac { y }{ x log x }\) = \(\frac { sin 2x }{ log x }\)
Solution:
The given differential equation may be written as
\(\frac { dy }{ dx }\) + \(\frac { 1 }{ x log x }\)y = \(\frac { sin 2x }{ log x }\)
This is of the form \(\frac { dy }{ dx }\) + Py = Q
Where P = \(\frac { 1 }{ x log x }\); Q = \(\frac { sin 2x }{ log x }\)
Thus, the differential equation is linear.
I.F = e^∫pdx
= e^{∫ \(\frac { 1 }{ x log x }\) dx}
= e^{∫ \(\frac { 1 }{ t }\) dt}
= e^{log t}
= log x
So, the solution of the given differential equation is
y × I.F = ∫ (Q × I.F) dx + c
y × log x = ∫ \(\frac { sin 2x }{ log x }\) × log x dx + c
= ∫ sin 2x dx + c
y log x = \(\frac { -cos 2x }{ 2 }\) + c
y log x + \(\frac { cos 2x }{ 2 }\) = c is a required solution.

Question 11.
(x + a) \(\frac { dy }{ dx }\) – 2y = (x + a)⁴
Solution:

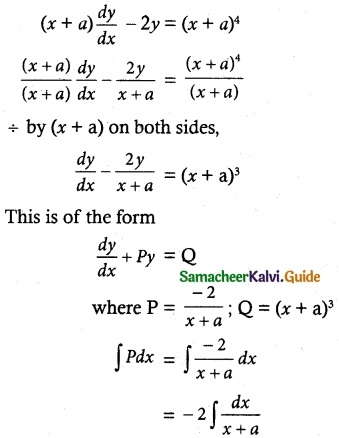
= -2 log (x + a)
= log (x + a)^-2

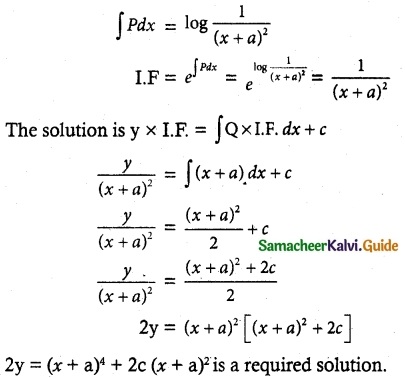
Question 12.
\(\frac { dy }{ dx }\) = \(\frac { sin^x }{ 1+x^3 }\) – \(\frac { 3x^2 }{ 1+x^3 }\)y
Solution:
The equation can be written as

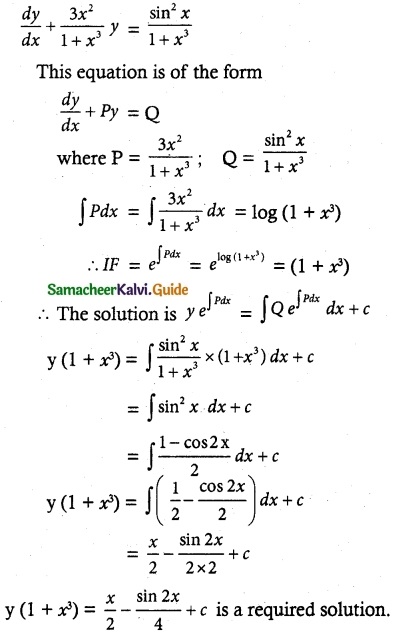
Question 13.
x \(\frac { dy }{ dx }\) + y = x log x
Solution:
The given differential equation may be written as

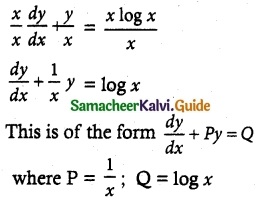
Thus, the given differential equation is linear.
I.F = e^∫pdx = e^{∫\(\frac { 1 }{ x }\) dx} = e^{log x} = x
So, the solution of the given differential equation is given by
y × I.F = ∫(Q × I.F)dx + c
yx = ∫ log x x dx + c
yx = ∫ x log x dx + c
u = log x ∫dv = ∫x dx

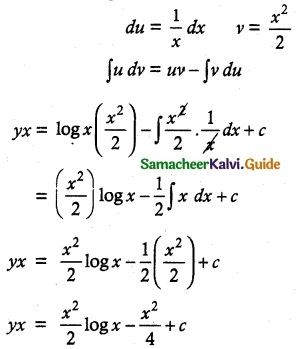
Multiply by 4
4yx = 2x² log x – x² + 4c
4xy = 2x² log x – x² + 4c is a required solution.

Question 14.
x \(\frac { dy }{ dx }\) + 2y – x² log x = 0
Solution:
The given differential equation may be written as
\(\frac { x }{ x }\) \(\frac { dy }{ dx }\) + \(\frac { 2y }{ x }\) = \(\frac { x log x }{ x }\)
This is of the form \(\frac { dy }{ dx }\) + Py = Q
where P = \(\frac { 2 }{ x }\); Q = x log x
Thus, the given equation is linear.
I.F = e^∫pdx = e^{∫\(\frac { 2 }{ x }\) dx}
e^{log x} = e^{log x²} = x²
So the required solution is
y × I.F = ∫(Q × I.F) dx + c
yx² = ∫x log x x² dx + c
yx² = ∫x³ logx dx + c

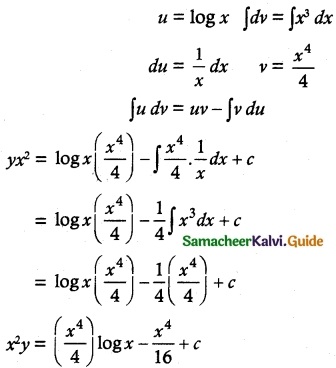
Multiply by 16
16x²y = 4x⁴ log x – x⁴ + 16c is a required solution

Question 15.
\(\frac { dy }{ dx }\) + \(\frac { 3y }{ x }\) = \(\frac { 1 }{ x^2 }\), given that y = 2 when x = 1
Solution:
The given differential equation can be written as

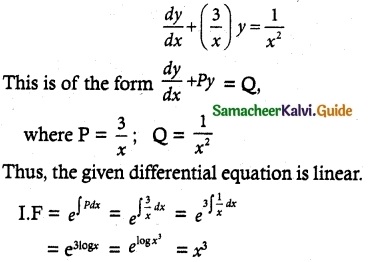
So, its solution is given by
y × I.F = ∫(Q × I.F) dx + c
yx³ = ∫ \(\frac { 1 }{ x^2 }\) + x³ dx + c
= ∫x dx + c
y x³ = \(\frac { x^2 }{ 2 }\) + c
2yx³ = x² + c
Given that y = 2 when x = 1
2 (2) (1)³ = 1 + c
4 – 1 = c
c = 3
∴ 2yx³ = x² + 3 is a required solution.