Samacheer Kalvi 11th Physics Guide Chapter 2 Kinematics

Tamilnadu State Board New Syllabus Samacheer Kalvi 11th Physics Guide Pdf Chapter 2 Kinematics Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 11th Physics Solutions Chapter 2 Kinematics

11th Physics Guide Kinematics Book Back Questions and Answers

Part – I:
I. Multiple choice questions:

Question 1.
Which one of the following Cartesian coordinate systems is not followed in physics?

Answer:

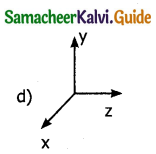
Question 2.
Identify the unit vector in the following _______.

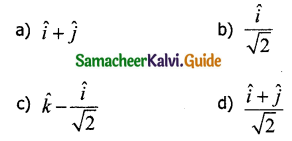
Answer:

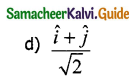
Question 3.
Which one of the following quantities cannot be represented by a scalar?
a) Mass
b) length
c) momentum
d) magnitude of the acceleration
Answer:
c) momentum

Question 4.
Two objects of masses m₁ and m₂ fall from the heights h₁ and h₂ respectively. The ratio of the magnitude of their momenta when they hit the ground is _______. (AIPMT 2012)

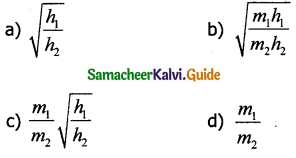
Answer:

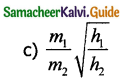
Question 5.
If a particle has negative velocity and negative acceleration, it speeds _______.
a) increases
b) decreases
c) remains the same
d) zero
Answer:
a) increases

Question 6.
If the velocity is \(\overline{V}\) = \(2 \hat{i}+t^{2} \hat{j}-9 \hat{k}\) then the magnitude of acceleration at t = 0.5s is _______.
a) 1ms^-2
b) 2 ms^-2
c) zero
d) -1ms^-2
Answer:
a) 1ms^-2

Question 7.
If an object is dropped from the top of a building and it reaches the ground at t = 4s, then the height of the building is (ignoring air resistance) (g = 9.8ms^-2)
a) 77.3m
b) 78.4m
c) 80.5
d) 79.2m
Answer:
b) 78.4m

Question 8.
A ball is projected vertically upwards with a velocity v. It comes back to the ground in time t. Which v-t graph shows the motion correctly? (NSEP 00-01)

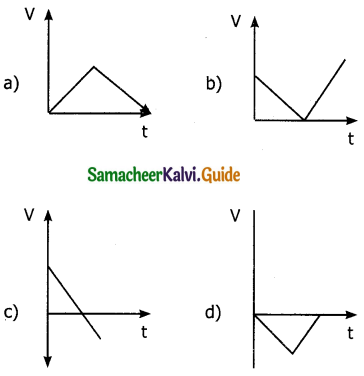
Answer:

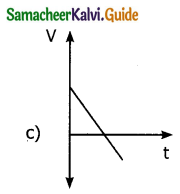
Question 9.
If one object is dropped vertically downward and another object is thrown horizontally from the same height, then the ratio of vertical distance covered by both objects at any instant t is _______.
a) 1
b) 2
c) 4
d) 0.5
Answer:
a) 1

Question 10.
A bail is dropped from some height towards the ground. Which one of the following represents the correct motion of the ball?

Answer:

Question 11.
If a particle executes uniform circular motion in the XY plane in a clockwise direction, then the angular velocity is in _______.
a) +y direction
b) +z direction
c) -z direction
d) -x direction
Answer:
c) -z direction

Question 12.
If a particle executes uniform circular motion, choose the correct statement _______. (NEET 2016)
a) The velocity and speed are constant.
b) The acceleration and speed are constant.
c) The velocity and acceleration are constant.
d) The speed and magnitude of acceleration are constant
Answer:
d) The speed and magnitude of acceleration are constant

Question 13.
If an object is thrown vertically up with the initial speed u from the ground, then the time taken by the object to return back to the ground is _______.
(a) \(\frac{u^{2}}{2 g}\)
(b) \(\frac{u^{2}}{g}\)
(c) \(\frac { u }{ 2g }\)
(d) \(\frac { 2u }{ g }\)
Answer:
(d) \(\frac { 2u }{ g }\)

Question 14.
Two objects are projected at angles 30° and 60° respectively with respect to the horizontal direction. The range of two objects are denoted as R_30° and R_60° Choose the correct relation from the following
a) R_30° = R_60°
b) R_30° = 4R_60°
c) R_30° = R_{\(\frac { 60° }{ 2 }\)}
d) R_30° = 2R_60°
Answer:
a) R_30° = R_60°

Question 15.
An object is dropped in an unknown planet from a height of 50m, it reaches the ground in 2s. The acceleration due to gravity in this unknown planet is _______.
a) g = 20ms^-2
b) g = 25ms^-2
c) g = 15ms^-2
d) g = 30ms^-2
Answer:
b) g = 25ms^-2

II. Short Answer Questions:

Question 1.
Explain what is meant by the Cartesian coordinate system?
Answer:
At any given instant of time, the frame of reference with respect to which the position of the object is described in terms of position coordinates (x,y,z) is called “Cartesian coordinate system”.

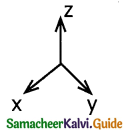
If x, y, and z axes are drawn in an anticlockwise direction, then the coordinate system is called a right-handed Cartesian coordinate system.

Question 2.
Define a vector. Give Example.
Answer:
Vector is a quantity which is described by both magnitude and direction. Geometrically a vector is a directed line segment.
Example – force, velocity, displacement.

Question 3.
Define a Scalar. Give Examples.
Answer:
Scalar is a property of a physical quantity which can be described only by magnitude.
Example: Distance, Mass, Temperature, Speed, Energy, etc.

Question 4.
Write short note on the scalar product between two vectors.
Answer:
The Scalar product of two vectors (dot product) is defined as the product of the magnitudes of both the vectors and the cosine of angle between them.
If \(\vec{A}\) and \(\vec{B}\) are two vectors having an angle θ between them, then their scalar or dot product is

Example: W = \(\vec{F}\).\(\vec{dr}\). Work done is a scalar product of force \(\vec{F}\) and \(\vec{r}\)

Question 5.
Write a Short note on vector product between two vectors.
Answer:
The vector product or cross product of two vectors is defined as another vector having a magnitude equal to the product of the magnitudes of two vectors and the sine of the angle between them. The direction of the product vector is perpendicular to the plane containing the two vectors, in accordance with the right hand screw rule or right hand thumb rule. Thus, if\(\overrightarrow{\mathrm{A}}\) and \(\overrightarrow{\mathrm{B}}\) are two vectors, then their vector product is written as \(\overrightarrow{\mathrm{A}}\) × \(\overrightarrow{\mathrm{B}}\) which is a vector C defined by \(\overrightarrow{\mathrm{c}}\) = \(\overrightarrow{\mathrm{A}}\) x \(\overrightarrow{\mathrm{B}}\) = (AB sin 0) \(\hat{n}\)
The direction \(\hat{n}\) of \(\overrightarrow{\mathrm{A}}\) x \(\overrightarrow{\mathrm{B}}\) , i.e., \(\overrightarrow{\mathrm{c}}\) is perpendicular to the plane containing the vectors \(\overrightarrow{\mathrm{A}}\) and \(\overrightarrow{\mathrm{B}}\).

Question 6.
How do you deduce that two vectors are perpendicular?
Answer:
If the vector product of the two given vectors is having maximum magnitude.
i.e sinθ = 90°, [ (\(\vec{A}\) x \(\vec{B}\))Max = AB\(\hat{n}\) ] then the two vectors are said to be perpendicular.

Question 7.
Define Displacement and distance.
Answer:
Distance is the actual path length traveled by an object in the given interval of time during the motion. It is a positive scalar quantity. Displacement is the difference between the final and initial positions of the object in a given interval of time. It can also be defined as the shortest distance between these two positions of the object. It is a vector quantity.

Question 8.
Define velocity and speed.
Answer:
Velocity – Velocity is defined as the rate of change of position vector with respect to time (or) defined as the rate of change of displacement. It Is a vector quantity.

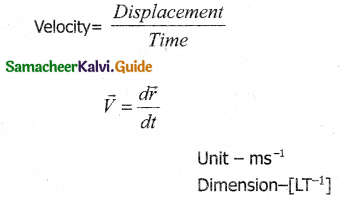
Speed – Speed is defined as the rate of change of distance. It is a scalar quantity.

Question 9.
Define acceleration.
Answer:
Acceleration is defined as the rate of change of velocity.
Acceleration \(\vec{a}\) = \(\frac{d \vec{v}}{d t}\)
Acceleration is a vector quantity.
Unit – ms^-2
Dimensional formula-[LT^-2]

Question 10.
What is the difference between velocity and average velocity?
Answer:

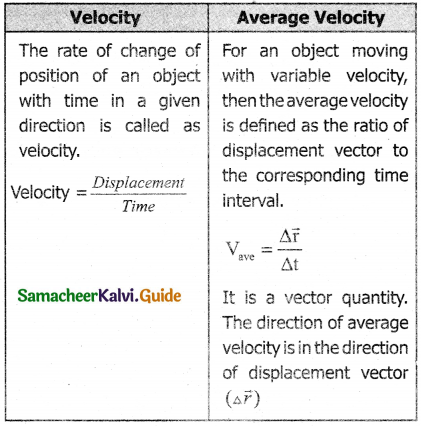
Question 11.
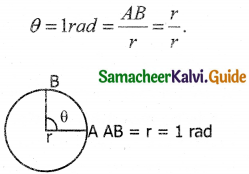
Define a radian.
Radian is defined as ratio of length of the arc to radians of the arc. One radian is the angle subtended at the center of the circle by an arc that is equal to in length to the radius of the circle.

Question 12.
Define angular displacement and angular velocity.
Answer:

1. Angular displacement: The angle described by the particle about the axis of rotation in a given time is called angular displacement.
2. Angular velocity: The rate of change of angular displacement is called angular velocity.

Question 13.
What is non-uniform circular motion?
Answer:
When an object is moving in a circular path with variable speed, it covers unequal distances in equal intervals of time. Then the motion of the object is said to be a non-uniform circular motion. Here both speed and direction during circular motion change.

Question 14.
Write down the kinematic equations for angular motion.
Answer:
The Kinematic equations for angular motion are ω = ω₀ + αt
θ = ω₀t + \(\frac { 1 }{ 2 }\)αt²
ω² = ω₀² + 2αθ
θ = \(\left(\frac{\omega_{0}+\omega}{2}\right)\) x t
ω₀ → initial angular velocity
ω → final angular velocity
α → angular acceleration
θ → angular displacement
t → time interval

Question 15.
Write down the expression for angle made by resultant acceleration and radius vector in the non-uniform circular motion.
Answer:
In the case of non-uniform circular motion, the particle will have both centripetal and tangential acceleration. The resultant acceleration is obtained as the vector sum of both centripetal and tangential acceleration.

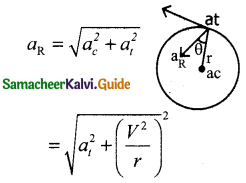
This resultant acceleration makes an angle 6 with a radius vector, which is given by

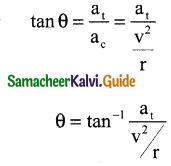
III. Long Answer Questions:

Question 1.
Explain in detail the triangle law of addition.
Answer:
Let us consider two vector \(\vec{A}\) and \(\vec{B}\) as shown In fig.

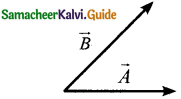
Law: To find the resultant of two vectors, the triangular law of addition can be applied as follows.
A and B are represented as the two adjacent sides of a triangle taken in the same order. The resultant is given by the third side of the triangle taken in reverse order.

Magnitude of the resultant vector:
from figure
Let θ be the angle between two vectors.
from ∆ ABN, Sin θ = \(\frac { BN }{ AB }\) ⇒ ∴ BN = B sinθ
Cos θ = \(\frac { AN }{ AB }\) ⇒ ∴ AN = B Cos θ

Which is the magnitude of the resultant \(\vec{A}\) and \(\vec{B}\).

The direction of the resultant vector:
If \(\vec{R}\) makes an angle α with \(\vec{A}\) then

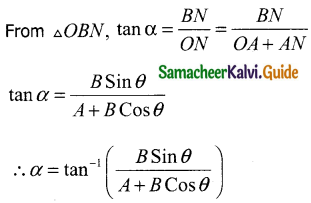
Question 2.
Discuss the properties of scalar and vector products.
Answer:
Properties of scalar product:
formula : \(\vec{A}\).\(\vec{B}\) = ABCosθ

1. The product quantity \(\overline{A}\).\(\overline{B}\) is always a scalar. It is positive if the angle between the vectors is acute (θ< 90°) and negative if angle between them is obtuse (90 < θ < 180)

2. The scalar product is commutative \(\overline{A}\).\(\overline{B}\) = \(\overline{B}\).\(\overline{A}\)

3. The scalar product obey distributive law. \(\overline{A}\).( \(\overline{B}\) + \(\overline{C}\) ) = \(\overline{A}\).\(\overline{B}\) + \(\overline{A}\).\(\overline{C}\)

4. The angle between the vector is θ = Cos^-1\(\frac{\bar{A} \cdot \bar{B}}{A B}\)

5. The scalar product of two vectors will be maximum when cos θ = 1 i.e θ = 0 ie when they are parallel.
[ ( \(\overline{A}\).\(\overline{B}\) ) max = AB.]

6. The scalar product of two vectors will be minimum when cos θ = -1 ie θ = 180°
( \(\overline{A}\).\(\overline{B}\))_mm = – AB [the vector are anti-parallel]

7. If two vector \(\overline{A}\) & \(\overline{B}\) are perpendicular to each other then \(\overline{A}\).\(\overline{B}\) = O. Because cos 90 = 0. Then vectors A & B are mutually orthogonal.

8. The scalar product of a vector with it self is termed as self or dot product and is given by
( \(\overline{A}\) )² = \(\overline{A}\).\(\overline{A}\) = AA cos θ = A²
Here 0=0
The magnitude or norm of the vector \(\overline{A}\) is
|A| = A = \(\sqrt{\bar{A} \cdot \bar{A}}\) = A.

9. Incase of orthogonal unit vectors
\(\hat{n}\).\(\hat{n}\) = 1 x 1cos0 = 1
for eg \(\hat{i}\).\(\hat{i}\) = \(\hat{j}\).\(\hat{j}\) = \(\hat{k}\).\(\hat{k}\) = 1

10. Incase of orthogonal unit vectors \(\hat{i}\), \(\hat{f}\), \(\hat{k}\) then \(\hat{i}\).\(\hat{j}\) = \(\hat{j}\).\(\hat{k}\) = \(\hat{k}\).\(\hat{j}\) = 1.1 cos 90 = 0.

11. In terms of components the scalar product of A and B can be written as

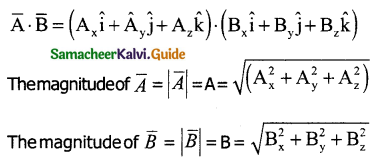
Properties of cross product:
Formula \(\vec{A}\) x \(\vec{B}\) = ABsinθ

1. The vector product of any two vectors is always an another vector whose direction perpendicular to the plane containing these two vectors, ie. Orthogonal to \(\overline{A}\) & \(\overline{B}\) even though \(\overline{A}\) & \(\overline{B}\) may not be mutually orthogonal.

2. Vector product is not commutative
\(\overline{A}\) x \(\overline{B}\) = – \(\overline{B}\).\(\overline{A}\)
\(\overline{A}\) x \(\overline{B}\) ≠ \(\vec{B}\) x \(\vec{A}\)
Here magnitude | \(\overline{A}\) x \(\overline{B}\) | = | \(\overline{B}\).\(\overline{A}\) | are equal but opposite direction.

3. The vector product of two vector is maximum when sine = 1, ie θ = 90°
ie. when \(\overline{A}\) and \(\overline{B}\) are orthogonal to each other.
( \(\overline{A}\) x \(\overline{B}\) ) max = AB \(\hat{n}\).

4. The vector product of two non zero vectors is minimum if |sinθ| = 0. ie. θ = 0 or 180°
( \(\overline{A}\) x \(\overline{B}\) ) m in = 0
Vector product of two non zero vectors is equal to zero if they either parallel or anti parallel

5. The self cross product ie product of a vector with itself is a null vector \(\overline{A}\) x \(\overline{B}\) = AA sinθ = 0

6. The self-vector product of the unit vector is zero
i.e. \(\hat{i}\).\(\hat{j}\) = \(\hat{j}\).\(\hat{j}\) = \(\hat{k}\).\(\hat{k}\) = 0

7. In case of orthogonal unit vectors \(\hat{i}\), \(\hat{j}\), \(\hat{k}\) in accordance with right hand cork screw rule \(\hat{i}\).\(\hat{j}\) =\(\hat{k}\), \(\hat{i}\).\(\hat{k}\) = \(\hat{i}\), \(\hat{k}\).\(\hat{i}\) = \(\hat{j}\) also since cross product is not commutative

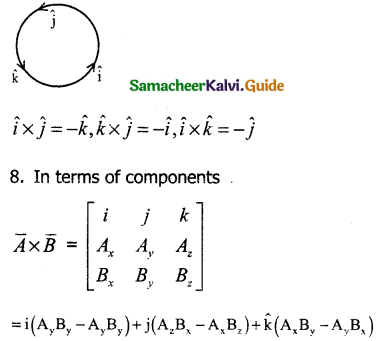
9. If two vectors \(\overline{A}\) & \(\overline{B}\) form adjacent sides of a parallelogram then the magnitude of |\(\overline{A}\) x \(\overline{B}\)| will give area 0f parallelogram.

10. Since one can divide a parallelogram into two equal triangles, the area of the triangle is \(\frac { 1 }{ 2 }\) |\(\overline{A}\) x \(\overline{B}\)|.

Question 3.
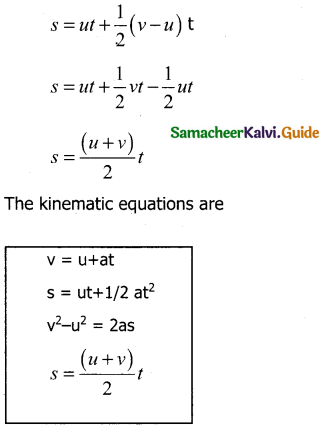
Derive the kinematic equations of motion for constant acceleration.
Answer:
Consider an object moving in a straight line with uniform or constant acceleration ‘a’. Let u be the initial velocity at t = 0, and v be the final velocity after a time of t seconds

(i) Velocity time relation:
The acceleration of the body at any instant is given by first derivative of the velocity with time
a = \(\frac { dv }{ dt }\)
dv = adt
integrating both sides

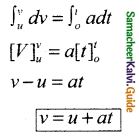
Displacement time relation:

(ii) The velocity of the body is given by the first derivative of the displacement with respect to time
But v = ds/dt
∴ dv = v dt
v = u + at
ds = (u + at)dt
ds = udt + atdt
Integrating both sides

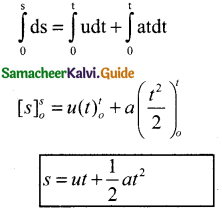
Velocity-displacement relation:

also we can derive from the relation v = u + at
v – u = at
Substituting in equation s = ut + \(\frac { 1 }{ 2 }\)at²

Question 4.
Derive the equations of motion for a particle (a) falling vertically (b) projected vertically.
Answer:
For a body falling vertically from a height ‘h’:
Consider an object of mass m falling from height h.
Neglecting air resistance, the downward direction as the positive y-axis.
The object experiences acceleration ‘g’ due to gravity which is constant near the surface of the earth.
In kinematic equations of motion \(\vec{a}\) = g\(\hat{i}\)
By comparing the components a_x = 0, a_g = 0, a_y= g

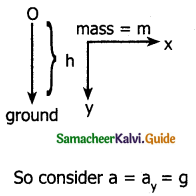
Case – 1
If the particle is thrown with initial velocity ‘u’ downward then
v = u + gt
y = ut + 1/2gt²
v² – u² = 2gy

Case – 2
Suppose the particle starts from rest,
u = 0
v = gt
y = 1/2gt²
v² = 2gy
For a body projected vertically: Consider an object of mass m thrown vertically upwards with an initial velocity u. Ne-glect air friction. The vertical direction as positive y axis then the acceleration,
a = – g
The kinematic equation of motion are v = u – gt
v = u – gt
s = ut – 1/2 gt²

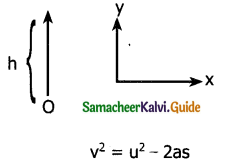
Question 5.
Derive the equations of motion, range, and maximum height reached by a particle thrown at an oblique angle θ with respect to the horizontal direction.
Answer:
Consider an object thrown with an initial velocity u at an angle θ with horizontal.

Then initial velocity is resolved into two components
u_x = u cos θ horizontally and
u_y = u sin θ vertically
At maximum height u_y = 0 (since acceleration due to gravity is opposite to the direction of the vertical component).
The Horizontal component of velocity
u_x = u cos θ remains constant throughout its motion.
hence after the time t the velocity along the horizontal motion
V_x = U_x + a_xt
= ux = cos θ
The horizontal distance travelled by the projectile in a time ‘t’ is S_x = u_xt + 1/2 a_xt².
Here S_x = x u_x = u cos θ
a_x = 0
∴ x = u cos θt ____ (1)
∴ t = \(\frac { x }{ u cos θ }\) ____ (2)
For vertical motion
V_y = u_y + a_yt
Here v_y = v_y
u_y = u sin θ
a_y = – g
v_y = u sin θ – gt
The vertical distance travelled by the projectile in the same time ‘t’ is
S_y = U_y t + a_y t²
S_y = y, U_y = u sin θ a_y = – g
y = u sin θ t – 1/2 gt² ____ (4)
Substituting the value of t in (4) we get equation:

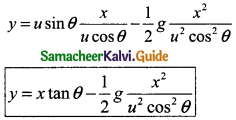
Which indicates the path followed by the projectile is an inverted parabola.

Expression for Maximum height:
The maximum vertical distance travelled by the projectile during its motion is called maximum height.
We know that
v_y² = u_y² + 2a_ys
Here u_y = u sin 0, a_y = – g, s = h_max
v_y = 0

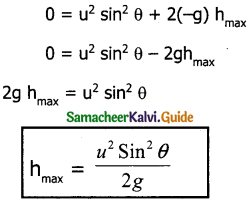
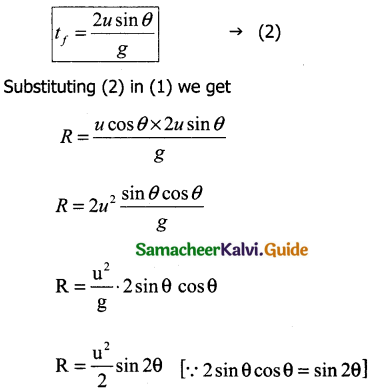
Expression for horizontal range:
The maximum horizontal distance between the point of projection and the point on the horizontal plane where the projectile hits the ground is called horizontal range.
Horizontal range = Horizontal component of velocity x time of flight
R = u cos θ x t_f → (1)
Time of flight (t_f) is the time taken by the projectile from point of projection to point the projectile hits the ground again
w.k.t = S_y = u_y t_f + 1/2 a_y t²_f)
Here S_y = 0 u_y = u sin θ, a_y = – g
0 = u sin θ t_f – 1/2g t²_f
1/2 gt t²_f = u sin θ t_f

Question 6.
Derive the expression for centripetal acceleration.
Answer:
In uniform circular motion the velocity vector turns continuously with out changing its magnitude. ie speed remains constant and direction changes. Even though the velocity is tangential to every point is a circle, the acceleration it acting towards the centre of the circle along the radius. This is called centripetal acceleration

Expression:
The centripetal acceleration is derived from a simple geometrical relationship between position and velocity vectors. Let the directions of position and velocity vectors shift through same angle θ in a small time interval ∆t
For uniform circular motion r = \(\left|\bar{r}_{1}\right|\) = \(\left|\bar{r}_{2}\right|\)
and v = \(\left|\bar{v}_{1}\right|\) = \(\left|\bar{v}_{2}\right|\)
If the particle moves from position vector \(\bar{r}_{1}\) to \(\bar{r}_{2}\) the displacement is given by \(\overrightarrow{\Delta r}\) = \(\bar{r}_{2}\) – \(\bar{r}_{1}\)

and change in velocity from \(\bar{v}_{1}\) to \(\bar{v}_{2}\) is given ∆\(\bar { v }\) = \(\bar{v}_{2}\) – \(\bar{v}_{1}\)

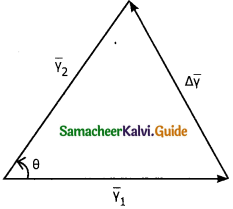
The magnitudes of the displacement ∆r and ∆v satisfy the following relation

Here negative sign indicates that ∆v points radially inwards, towards the centre of the circle

For uniform circular motion v=rω where ω is the angular velocity of the particle about the center
The centripetal acceleration a = ω²r.

Question 7.
Derive the expression for total acceleration in the non-uniform circular motion.
Answer:
If the velocity changes both in speed and direction during circular motion, then we get non-uniform circular motion. Whenever the speed is not the same in a circular motion then the particle will have both centripetal and tangential acceleration.

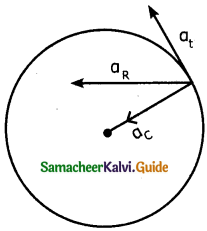
The resultant acceleration is obtained by the vector sum of centripetal and tangential acceleration
Let the tangential acceleration be a_t.
Centripetal acceleration is v²/r.
The magnitude of the resultant acceleration is a_R = \(\sqrt{a_{t}^{2}+\left(\frac{v^{2}}{r}\right)^{2}}\)

IV. Exercises:

Question 1.
The position vector particle has a length of 1m and makes 30° with the x-axis what are the lengths of x and y components of the position vector?
Solution:

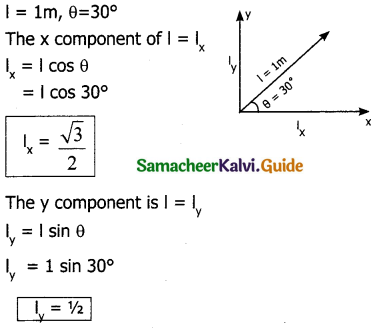
Question 2.
A particle has its position moved from \(\left|\bar{r}_{1}\right|\) = 3\(\hat{i}\) + 4\(\hat{j}\) to r\(\left|\bar{r}_{2}\right|\) = \(\hat{i}\)+ 2\(\hat{j}\) calculate the displacement vector ( ∆ \(\vec{r}\) ) and draw the \(\left|\bar{r}_{1}\right|\), \(\left|\bar{r}_{2}\right|\) and ( ∆ \(\vec{r}\) ) vector in a two dimensional Cartesian co-ordinate system.
Solution:

Question 3.
Calculate the average velocity of the particle whose position vector changes from \(\left|\bar{r}_{1}\right|\) = 5\(\hat{i}\) + 6\(\hat{j}\) to \(\left|\bar{r}_{2}\right|\) = 2\(\hat{i}\) + 3\(\hat{j}\) in a time 5 seconds.
Solution:

Question 4.
Convert the vector \(\overline{r}\) = 3\(\hat{i}\) + 2\(\hat{j}\) into a unit vector.
Solution:
A vector divided by its magnitude is a unit vector

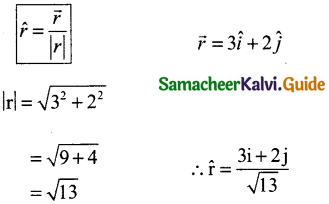
Question 5.
What are the resultants of the vector product of two given vector given by
\(\overline{A}\) = 4\(\hat{i}\) – 2\(\hat{j}\) + \(\hat{k}\) and \(\overline{B}\) = 5\(\hat{i}\) + 3\(\hat{j}\) – 4\(\hat{k}\)?
Solution:

Question 6.
An object at an angle such that the horizontal range is 4 times the maximum height. What is the angle of projection of the object?
Solution:
Incase of obliging projection

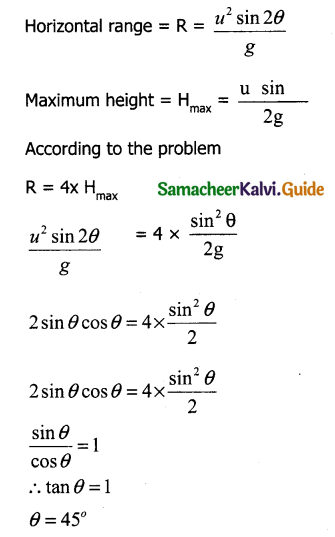
Question 7.
The following graphs represent velocity-time graph. Identify what kind of motion a particle undergoes in each graph.

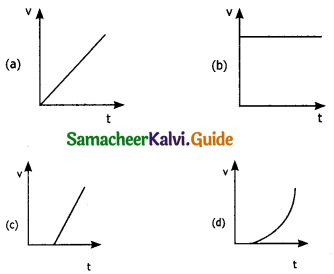
Solution:
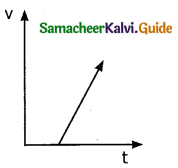
(a) When the body starts from rest and moves with uniform acceleration is constant

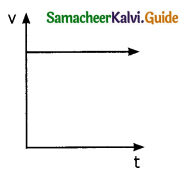
(b) This graph represents, for a body moving with a uniform velocity or constant velocity. The zero slope of curve indicates zero acceleration.

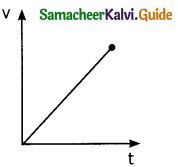
(c) This v-t graph is a straight line not passing through origin indicates the body has a constant acceleration but greater than fig(i) as slope is more than the first one (more steeper)

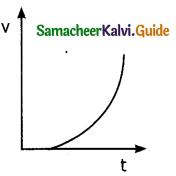
(d) Greater changes in velocity (velocity variations are taking place in equal as travels of time. The graph indicates increasing acceleration.

Question 8.
The following velocity-time graph represents a particle moving in the positive x-direction. Analyse its motion from o to 7s calculate the displacement covered and distance traveled by the particle from 0 to 2s.

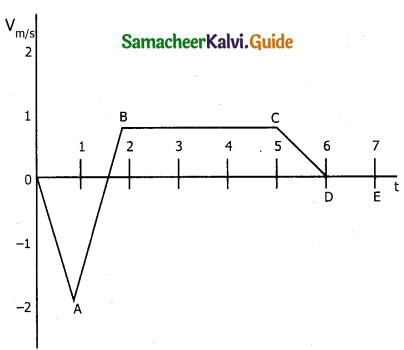
Solution:
From o to A(o to Is):
At t = os the particle has a zero velocity at t > 0 the particle has a negative velocity and moves in positive x-direction the slope dr/dt is negative. The particle is decelerating. Thus the velocity decreases during this time interval.

From A to B (Is to 2s):
From time Is to 2s the velocity increase and slope dv/dt becomes positive. The particle is accelerating. The velocity increases in this time interval.

From B to C (2s to 5s):
From 2s to 5s the velocity stays constant at 1 m/s. The acceleration is zero.

From C to D (6s to 7s):
From 5s to 6s the velocity decreases. Slope dv/dt is negative. The particle is decelerating. The velocity decreases to zero. The body comes to rest at 6s.

From D to E (6s to 7s)
The particle is at rest during this time interval.

Displacement: in 0 – 2s:
The total area under the curve from 0 to 2s displacement = 1/2bh + 1/2bh
=1/2 x 1.5 x (- 2) + 0.5 x 1
= – 1.5 + 0.25
= – 1.25 m

Distance: is 0 – 2s
The distance covered is = 1.5 + 0.25 = 1.75 m

Question 9.
A particle is projected at an angle of θ with respect to the horizontal direction. Match the following for the above motion.
(a) v_x – decreases and increases
(b) V_y – remains constant
(c) Acceleration – varies
(d) Position vector – remains downwards
Solution:
(a) V_x – remains constant
(b) V_y – decreases and increases
(c) Acceleration (a) – remains downwards
(d) Position vector (r) – varies

Question 10.
A water fountain on the ground sprinkles water all around it. If the speed of the water coming out of the fountains is V. Calculate the total area around the fountain that gets wet.
Solution :
Speed of water = V
(Range)_max = radius = u₂/g = v²/g
This range becomes the radius = (v²/g) of the circle where water sprinkled.
Area covered = Area of circle
= πr² = π\(\left(\frac{v^{2}}{g}\right)\)²
= π \(v^{4} / g^{2}\)

Question 11.
The following table gives the range of the particle when thrown on different planets. All the particles are thrown at the same angle with the horizontal and with the same initial speed. Arrange the planets in ascending order according to their acceleration due to gravity (g value)

Solution:
R = – (sin 2θ)
∵ the initial velocity and angle of projection are constants
R ∝ \(\frac { 1 }{ g }\)
g ∝ \(\frac { 1 }{ R }\)

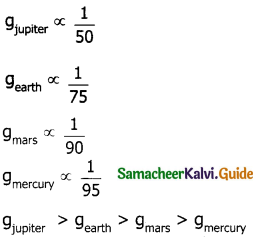
According to acceleration, due to gravity In ascending order, the solution is. Mercury, Mars, Earth, Jupiter

Question 12.
The resultant of two vectors A and B is perpendicular to vector A and its magnitude is equal to half of the magnitude of vector B. Then the angle between A and B is
(a) 30°
(b) 45°
(c) 150°
(d)120°
Solution:
Let two vectors be A & B

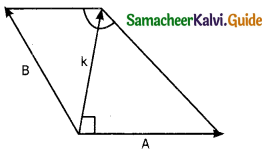
Magnitude of B = B
Magnitude of A = A
∝ = 90°
Given:

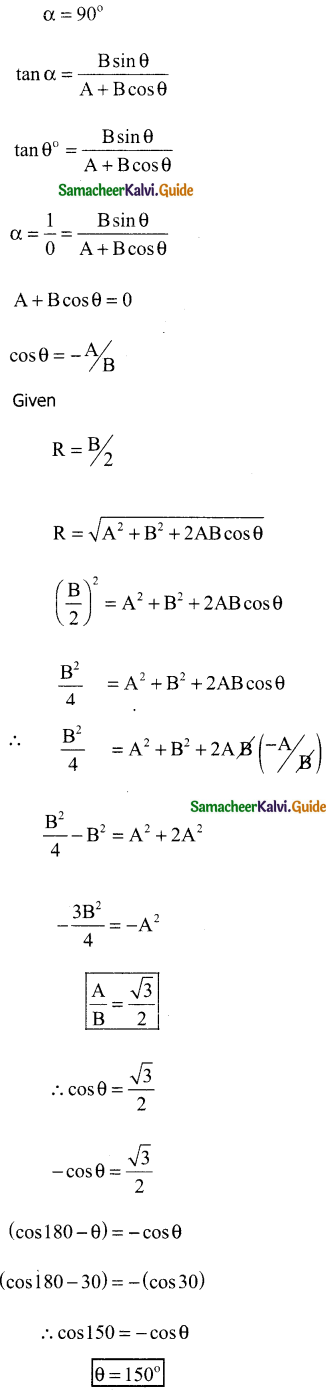
Question 13.
Compare the components for the following vector equations.
(a) T\(\hat{j}\) – mg\(\hat{j}\) = ma\(\hat{j}\)
(b) \(\overline{T}\) + \(\overline{F}\) = \(\overline{A}\) + \(\overline{B}\)
(c) \(\overline{T}\) – \(\overline{F}\) = \(\overline{A}\) – \(\overline{B}\)
(d) T\(\hat{j}\) + mg\(\hat{j}\) = ma\(\hat{j}\)
Solution:
We can resolve all vectors in x, y, z components w.r.t. Cartesian co-ordinate system. After resolving the components separately equate x components on both sides y components on both sides and z components on both side we get.
(a) T\(\hat{j}\) – mg\(\hat{j}\) = ma\(\hat{j}\)
T – mg = ma

(b) \(\overline{T}\) + \(\overline{F}\) = \(\overline{A}\) + \(\overline{B}\)
T_x + F_x = A_x + B_x

(c) \(\overline{T}\) – \(\overline{F}\) = \(\overline{A}\) – \(\overline{B}\)
T_x – F_x = A_x – B_x

(d) T\(\hat{j}\) + mg\(\hat{j}\) = ma\(\hat{j}\)
T + mg = ma

Question 14.
Calculate the area of the triangle for which two of its sides are given by the vectors \(\overline{A}\) = 5\(\hat{i}\) – 3\(\hat{j}\) \(\overline{B}\) = 4\(\hat{i}\) + 6\(\hat{j}\).
Solution:

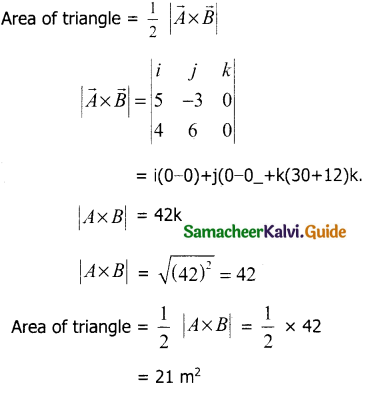
Question 15.
If the earth completes one revolution in 24 hours, what is the angular displacement made by the earth in one hour? Express your answer in both radian and degree.
Solution:
ω = θ/t θ = wt
In 24 hours, angular displacement made
θ = 360° (or) 2π rad
In 1 hours, angular displacement
θ = \(\frac { 360° }{ 24 }\)
θ = 15°
In radian θ = \(\frac { 2π }{ 24 }\) = \(\frac { π }{ 12 }\) radians.
θ = \(\frac { π }{ 12 }\) rad.

Question 16.
An object is thrown with initial speed of 5ms^-1 with an angle of projection of 30°. What is the height and range reached by the particle?
Solution:
u = 5 m/s
θ = 30°
h_max = ?
R = ?
Height reached

Range:

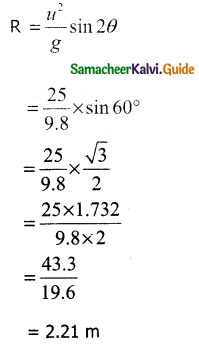
Question 17.
A football player hits the ball with a speed 20m/s with angle 30° with respect to as shown in the figure horizontal directions. The goal post is at a distance of 40 m from him. Find out whether the ball reaches the goal post.

Solution :
In order to find whether the ball is reaching the goal post the range should be equal to 40m so range

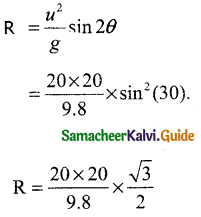
= \(\frac { 692.8 }{ 19.6 }\)
= 35.35 m.
Which is less than the distance of the goal post which is 40 m away so the ball won’t reach the goal post.

Question 18.
If an object is thrown horizontally with an initial speed 10 ms^-1 from the top of a building of height 100 m. What is the horizontal distance covered by the particle?
Solution:
u = 10 m/s
h = 100 m
x = ?
x = u x T

x = 45.18 m.

Question 19.
An object is executing uniform circular motion with an angular speed of π/12 radians per second. At t = 0 the object starts at an angle θ = 0. What is the angular displacement of the particle after 4s?
Solution :
ω = π/12 rad/s
ω = θ/t
θ = w x t = π/12 x 4
θ = π/3 radian
θ = \(\frac { 180° }{ 3 }\)
= 60°

Question 20.
Consider the x-axis as representing east, the y-axis as north, and the z-axis as vertically upwards. Give the vector representing each of the following points.

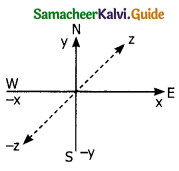
(a) 5m northeast and 2m up.
(b) 4m southeast and 3m up.
(c) 2m northwest and 4m up.
Solution:
5m northeast and 2m up.
(a) The vector representation of 5m N-E and 2m up is (5i + 5j) Cos 45° + 2\(\hat{k}\)

(b) 4m south east and 3m up.

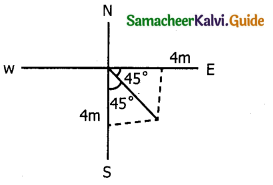
The vector representing 4m south east and 3m up is
(4i – 4j) cos 45 + 3\(\hat{k}\)
\(\frac{4(i-j)}{\sqrt{2}}\) + 3\(\hat{k}\)

(c) 2m north west and 4m up.

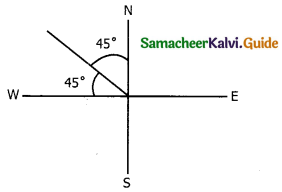
The vector representing 2m northwest and 4m up

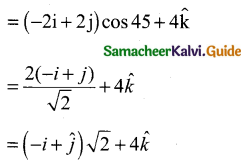
Question 21.
The moon is orbiting the earth approximately once in 27 days. What is the angle transversed by the moon per day?
Solution :
Angle described in 27 days = 2π rad = 360° days
Angie described in one day = 2π/27 radian
= \(\frac { 360° }{ 27 }\)
θ = 13.3°

Question 22.
An object of mass m has an angular acceleration ∝ = 0.2 rad/s². What is the angular displacement covered by the object after 3 seconds? (Assume that the object started with angle zero with zero angular velocity)
Solution:
∝ = 0.2 rad/s²
θ = ? t = 3s.
w₀ = 0
w.k.T θ = ω₀t + 1/2 ∝ t²
θ = 0 + 1/2 x 0.2 x 9
θ = 0.9 rad
θ = 0 = 0.9 x 57.295° = 51°
The magnitude of the resultant vector R is given by

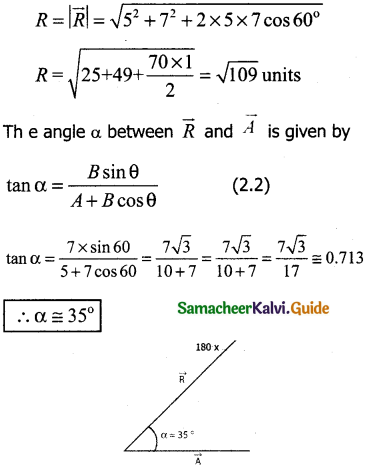
11th Physics Guide Kinematics Additional Important Questions and Answers

I. Multiple choice questions:

Question 1.
A particle moves in a circle of radius R from A to B as in the figure.

Answer:

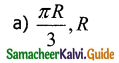
Question 2.
The branch of mechanics which deals with the motion of objects without taking force into account is –
(a) kinetics
(b) dynamics
(c) kinematics
(d) statics
Answer:
(c) kinematics

Question 3.
A particle moves in a straight line from A to B with speed v₁ and then from B to A with speed v₂. The average velocity and average speed are _______.

Answer:

Question 4.
A particle is moving in a straight line under constant acceleration. It travels 15m in the 3^rd second and 31m in the 7^th second. The initial velocity and acceleration are _______.
a) 5 m/s, 4 m/s²
b) 4 m/s, 5 m/s²
c) 4 m/s, 4 m/s²
d) 5 m/s, 5 m/s²
Answer:
a) 5 m/s, 4 m/s²

Question 5.
If an object is moving in a straight line then the motion is known as –
(a) linear motion
(b) circular motion
(c) curvilinear motion
(d) rotational motion
Answer:
(a) linear motion

Question 6.
A car is moving at a constant speed of 15 m/s. Suddenly the driver sees an obstacle on the road and takes 0.4 s to apply the brake. The brake causes a deceleration of 5 m/s². The distance traveled by car before it stops _______.
a) 6 m
b) 22.5 m
c) 28.5 m
d) 16.2 m
Answer:
c) 28.5 m

Question 7.
A car accelerates from rest at a constant rate for some time after which it decelerates at a constant rate (3 to come to rest. If the total time lapses in ‘t’ seconds, then the maximum velocity reached is _______.

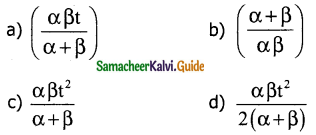
Answer:

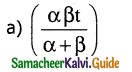
Question 8.
Spinning of the earth about its own axis is known as –
(a) linear motion
(b) circular motion
(c) curvilinear motion
(d) rotational motion
Answer:
(d) rotational motion

Question 9.
A particle is thrown vertically up with a speed of 40m/s, The velocity at half of the maximum height _______.
a) 20 m/s
b) 20\(\sqrt{2}\)m/s
c) 10 m/s
d) 10\(\sqrt{2}\)m/s
Answer:
b) 20\(\sqrt{2}\)m/s

Question 10.
The ratio of the numerical values of the average velocity and the average speed of the body is always _______.
a) unity
b) unity or less
c) unity or more
d) less than unity
Answer:
b) unity or less

Question 11.
The motion of a satellite around the earth is an example for –
(a) circular motion
(b) rotational motion
(c) elliptical motion
(d) spinning
Answer:
(a) circular motion

Question 12.
One car moving on a straight road covers one-third of the distance with 20 km/h and the rest with 60 km/h. The average speed is _______.
a) 40 km/h
b) 80km/h
c) 46\(\frac { 2 }{ 3 }\) km/hr
d) 36 km/h
Answer:
d) 36 km/h

Question 13.
A 150m long train is moving with a uniform velocity of 45 km/h. The time taken by the train to cross a bridge of length 850m is _______.
a) 56s
b) 68s
c) 80s
d) 92s
Answer:
c) 80s

Question 14.
A particle moves in a straight line with constant acceleration. It changes its velocity from 10 m/s to 20 m/s while passing through a distance of 135 m in ‘t’ seconds. The value of t is _______.
a) 12s
b) 9s
c) 10s
d) 1.8s
Answer:
b) 9s

Question 15.
If a ball is thrown vertically upwards with a speed u the distance covered during the last ‘t’ seconds of its ascent is _______.
a) 1/2 gt²
b) ut – 1/2gt²
c) (u – gt)t
d) ut
Answer:
a) 1/2 gt²

Question 16.
A particle moves along a straight line such that its displacement ‘s’ at any time ‘t’ is given by s = t³ – 6t² + 3t + 4 meters, t being in second. The velocity when acceleration is zero is _______.
a) 3 m/s
b) -12m/s
c) 42 m/s
d) -9 m/s
Answer:
d) – 9 m/s

Question 17.
Which of the following is not a scalar?
(a) Volume
(b) angular momentum
(c) Relative density
(d) time
Answer:
(b) angular momentum

Question 18.
Vector is having –
(a) only magnitude
(b) only direction
(c) bot magnitude and direction
(d) either magnitude or direction
Answer:
(c) both magnitude and direction

Question 19.
The displacement-time graph of a moving particle is shown below.

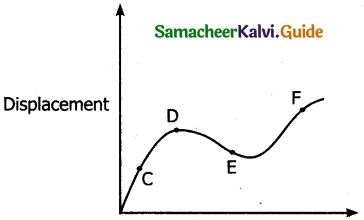
The instant velocity of the particle is negative at the point
a) D
b) F
c) C
d) E
Answer:
d) E

Question 20.
If two vectors are having equal magnitude and the same direction is known as –
(a) equal vectors
(b) col-linear vectors
(c) parallel vectors
(d) on it vector
Answer:
(a) equal vectors

Question 21.
The velocity-time graph of a body moving in a straight line is shown below _______.

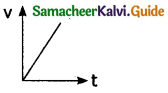
Which are of the following represents its acceleration-time graph?

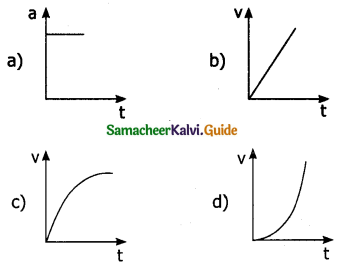
Answer:

Question 22.
Indicate which of the following graph represents the one-dimensional motion of particle?

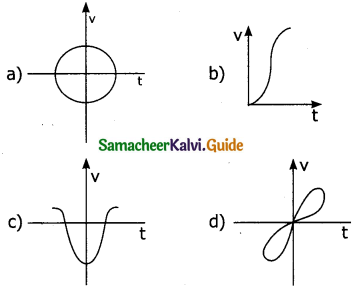
Answer:

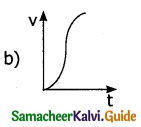
Question 23.
The variation of velocity of a particle with time moving along a straight line is illustrated in the following figure. The distance travelled by the particle in 4s is _______.

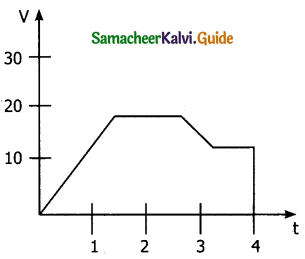
a) 60m
b) 55m
c) 25m
d) 30m
Answer:
b) 55m

Question 24.
An object is moving with a uniform acceleration which is parallel to its instantaneous direction of motion. The displacement (s), velocity (v) graph of this object is _______.

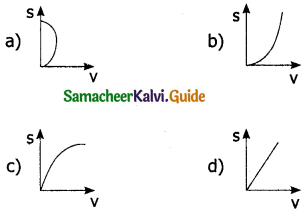
Answer:

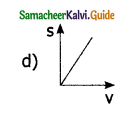
Question 25.
A unit vector is used to specify –
(a) only magnitude
(b) only direction
(c) either magnitude (or) direction
(d) absolute value
Answer:
(b) only direction

Question 26.
A vector is not changed if _______.
a) It is rotated through an arbitrary angle
b) It is multiplied by an arbitrary scalar
c) It is cross multiplied by a unit vector
d) It is parallel to itself.
Answer:
d) It is parallel to itself.

Question 27.
Two forces each of magnitude ‘F’ have a resultant of the same magnitude. The angle between two forces
a) 45°
b) 120°
c) 150°
d) 60°
Answer:
b) 120°

Question 28.
The magnitude of a vector can not be-
(a) positive
(b) negative
(e) zero
(cl) 90
Answer:
(b) negative

Question 29.
Six vectors \(\vec{a}\) through \(\vec{f}\) have magnitudes and directions as indicated in figure. Which of the following statement is true?

a) \(\overline{b}\) + \(\overline{e}\) = \(\overline{f}\)
b) 3\(\hat{i}\) – 2\(\hat{j}\) + \(\hat{k}\) \(\hat{b}\) + \(\hat{c}\) = \(\hat{f}\)
c) \(\hat{d}\) + \(\hat{c}\) = \(\hat{f}\)
d) \(\hat{d}\) + \(\hat{e}\) = \(\hat{f}\)
Answer:
d) \(\hat{d}\) + \(\hat{e}\) = \(\hat{f}\)

Question 30.
A force of 3 N and 4 N are acting perpendicular to an object, the resultant force is-
(a) 9 N
(b) 16 N
(c) 5 N
(d) 7 N
Answer:
(c) 5 N

Question 31.
The figure shows ABCDEF as regular hexagon. What is the value of
\(\overline{AB}\) + \(\overline{AC}\) + \(\overline{AD}\) + \(\overline{AE}\) + \(\overline{AF}\)?

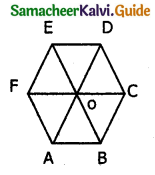
a) \(\overline{A0}\)
b) 2 \(\overline{A0}\)
c) 4 \(\overline{A0}\)
d) 6 \(\overline{A0}\)
Answer:
d) 6 \(\overline{A0}\)

Question 32.
One of the two rectangular components of a force is 20N. And it makes an angle of 30° with the force. The magnitude of the other component is _______.
a) 20/\(\sqrt{3}\)
b) 10/\(\sqrt{3}\)
c) 15/V\(\sqrt{3}\)
d) 40\(\sqrt{3}\)
Answer:
a) 20/\(\sqrt{3}\)

Question 33.
The angle between (\(\overrightarrow{\mathrm{A}}\) + \(\overrightarrow{\mathrm{B}}\)) and (\(\overrightarrow{\mathrm{A}}\) – \(\overrightarrow{\mathrm{B}}\)) can be –
(a) only 0°
(b) only 90°
(c) between 0° and 90°
(d) between 0° and 180°
Answer:
(d) between 0° and 180°

Question 34.
If the sum of two unit vectors is a unit vector the magnitude of the difference is _______.
a) \(\sqrt{2}\)
b) \(\sqrt{3}\)
c) 1/\(\sqrt{2}\)
d) \(\sqrt{5}\)
Answer:
b) \(\sqrt{3}\)

Question 35.
If P = mV then the direction of P along-
(a) m
(b) v
(c) both (a) and (b)
(d) neither m nor v
Answer:
(b) v

Question 36.
If \(\overline{A}\) = 2\(\hat{i}\) + \(\hat{j}\) – \(\hat{k}\), \(\overline{B}\) = \(\hat{i}\) + 2\(\hat{j}\) + 3\(\hat{k}\) and \(\overline{C}\) = 6\(\hat{i}\) – 2\(\hat{j}\) – 6\(\hat{k}\) then angle between \(\overline{A}\) + \(\overline{B}\) and \(\overline{C}\) will be _______.
a) 30°
b) 45°
c) 60°
d) 90°
Answer:
d) 90°

Question 37.
The scalar product \(\overrightarrow{\mathrm{A}}\).\(\overrightarrow{\mathrm{B}}\)is equal to-
(a) \(\overrightarrow{\mathrm{A}}\) +\(\overrightarrow{\mathrm{B}}\)
(b) \(\overrightarrow{\mathrm{A}}\). \(\overrightarrow{\mathrm{B}}\)
(c) AB sin θ
(d) (\(\overrightarrow{\mathrm{A}}\) x \(\overrightarrow{\mathrm{B}}\)
Answer:
(b) \(\overrightarrow{\mathrm{A}}\). \(\overrightarrow{\mathrm{B}}\)

Question 38.
If \(\overline{A}\) x \(\overline{B}\) = \(\overline{C}\) then which of the following statement is wrong?
a) \(\overline{C}\) ⊥\(\overline{A}\)
b) \(\overline{B}\) ⊥\(\overline{B}\)
c) \(\overline{C}\) ± ( \(\overline{A}\) + \(\overline{B}\) )
d) \(\overline{C}\) ± ( \(\overline{A}\) x \(\overline{B}\) )
Answer:
d) \(\overline{C}\) ± ( \(\overline{A}\) x \(\overline{B}\) )

Question 39.
The scalar product of two vectors will be minimum. When θ is equal to –
(a) 0°
(b) 45°
(c) 180°
(d) 60°
Answer:
(c) 180°

Question 40.
If | \(\overline{A}\) x \(\overline{B}\) |, then value of | \(\overline{A}\) x \(\overline{B}\) | is _______.

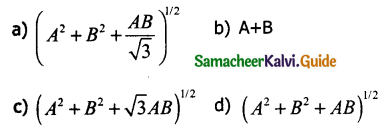
Answer:
d) (A² + B² + AB)\(\frac { 1 }{ 2 }\)

Question 41.
The angle between vectors \(\overline{A}\) and \(\overline{B}\) is A. The value of the triple product \(\overline{A}\) ( \(\overline{A}\) x \(\overline{B}\) ) is _______.
a) A² B
b) zero
c) A² B sinθ
d) A² B cos θ
Answer:
d) A² B cos θ

Question 42.
Two adjacent sides of a parallelogram are represented by the two vectors \(\hat{i}\) + 2\(\hat{j}\) + 3\(\hat{k}\) and 3\(\hat{i}\) – 2\(\hat{j}\) + \(\hat{k}\). The area parallelogram _______.
a) 8
b) 8\(\sqrt{3}\)
c) 3\(\sqrt{8}\)
d) 192
Answer:
b) 8\(\sqrt{3}\)

Question 43.
If \(\overrightarrow{\mathrm{A}}\) and \(\overrightarrow{\mathrm{B}}\) are two vectors, which are acting along x, y respectively, then \(\overrightarrow{\mathrm{A}}\) and \(\overrightarrow{\mathrm{B}}\) lies along-
(a) x
(b) y
(c) z
(d) none
Answer:
(c) z

Question 44.
Galileo writes that for angles of the projectile (45 + θ) and (45 – θ) the horizontal ranges described by the projectile are in the ratio of (if θ ≤ 45)
a) 2:1
b) 1:2
c) 1:1
d) 2:3
Answer:
c) 1:1

Question 45.
A projectile is thrown into the air so as to have the minimum possible range equal to 200. Taking the projection point as the origin the Coordinates of the point where the velocity of the projectile is minimum are _______.
a) 200,50
b) 100,50
c) 100,150
d) 100,100
Answer:
b) 100,50

Question 46.
\(\overrightarrow{\mathrm{A}}\) x \(\overrightarrow{\mathrm{B}}\) isequal to –
(a) \(\overrightarrow{\mathrm{B}}\) x \(\overrightarrow{\mathrm{A}}\)
(b) \(\overrightarrow{\mathrm{A}}\) + \(\overrightarrow{\mathrm{B}}\)
(c) –\(\overrightarrow{\mathrm{B}}\) x \(\overrightarrow{\mathrm{A}}\)
(d) \(\overrightarrow{\mathrm{A}}\) – \(\overrightarrow{\mathrm{B}}\)
Answer:
(c) –\(\overrightarrow{\mathrm{B}}\) x \(\overrightarrow{\mathrm{A}}\)

Question 47.
The vector product of any two vectors gives a –
(a) vector
(b) scalar
(e) tensor
(d) col-linear
Answer:
(a) vector

Question 48.
A 150 m long train is moving the north at a speed of 10 m/s. A parrot flying towards the south with a speed of 5 m/s crosses the train. The time taken would be _______.
a) 30s
b) 15s
c) 8s
d) 10s
Answer:
d) 10s

Question 49.
A boat is moving with a velocity of 3i+4j with respect to the ground. The water in the river is moving with a velocity of -3i-4j with respect to the ground. The relative velocity of the boat with respect to water _______.
a) 8j
b) -6i -8j
c) 6i + 8j
d) 5\(\sqrt{2}\)
Answer:
c) 6i + 8j

Question 50.
The vector product of two non-zero vectors will be minimum when O is equal to-
(a) 0°
(b) 180°
(e) both (a) and (b)
(d) neither (a) nor (b)
Answer:
(e) both (a) and (b)

II. Long Answer Questions:

Question 1.
What are the different types of motion? State one example for each & explain.
Answer:
The different types of motions are:
a) Linear motion: An object is said to be in linear motion if it moves in a straight line.
Example: An athlete running on a straight tack.

b) Circular motion: It is defined as a motion described by an object traveling a circular path.
Example: The motion of a satellite around the earth

C) Rotational motion: If any object moves in a rotational motion about an axis the motion is rotational motion. During rotation, every point in the object traverses a circular path about an axis.
Example: Spiring of earth about its own axis

D) Vibratory motion: If an object or a particle executes to and fro motion about the fixed point it is said to be in vibratory motion. Sometimes called oscillatory motion.
Example: Vibration of a string on a Guitar.

Question 2.
How will you differentiate motion in one dimension, two dimensions, and in three dimensions?
Answer:
Motion in one dimension: One-dimensional motion is the motion of a particle moving along a straight line.
Example: An object falling freely under gravity close to the earth.

Motion in two dimensions: If a particle is moving along a curved path in-plane, then it is said to be in two-dimensional motion.
Example: Motion of a coin in a carom board.

Motion in three dimensions: A particle moving in usual three-dimensional space has three-dimensional motion.
Example: A bird flying in the sky.

Question 3.
State and define different types of vectors.
Answer:
The different types of vectors are:
1. Equal vectors:
Two vectors \(\vec{A}\) & \(\vec{B}\) are said to be equal when they have equal magnitude and same direction and represent the same physical quantity.

(a) Coilinear vectors: Collinear vectors are those which act along the same line. The angle between them can be 0° or 180°

(i) Parallel vectors – If two vectors \(\vec{A}\) & \(\vec{B}\) act in the same direction along the same line or in parallel lines. Angle between them is equal to zero

(ii) Antiparallel vectors:
Two vectors \(\vec{A}\) & \(\vec{B}\) are said to be antiparallel when they are in opposite direction along the same line or in parallel lines. The angle between them is 180°

2. Unit vector:
A vector divided by its own magnitude is a unit vector.
The unit vector of \(\vec{A}\) is represented as \(\hat{A}\)
Its magnitude is equal to 1 or unity

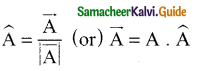
3. Orthogonal unit vectors:
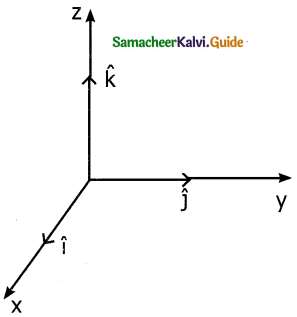
Let \(\hat{i}\), \(\hat{j}\), \(\hat{k}\) be three unit vectors which specify the direction along positive x-axis, positive y-axis and positive z-axis respectively. These three unit vectors are directly perpendicular to each other
The angle between any two of them is 90°. Then \(\hat{i}\), \(\hat{j}\), \(\hat{k}\) are examples of orthogonal vectors. Two vectors which are perpendicular to each other are called orthogonal vectors.

Question 4.
Explain how two vectors are subtracted when they are inclined to an angle θ.

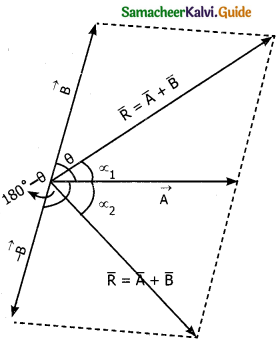
Let \(\overline{A}\) and \(\overline{B}\) be non zero vectors inclined at an angle θ.
The difference \(\overline{A}\) – \(\overline{B}\) can be obtained as follows.
First obtain – \(\overline{B}\)
The angle between \(\overline{A}\) – \(\overline{B}\)
= 180 – θ.
The difference \(\overline{A}\) – \(\overline{B}\) is the same as the resultant of \(\overline{A}\) – – \(\overline{B}\)

[∵ cos 180 – θ = – cos θ]
(cos 180 – θ = – cos θ)
The gives the resultant magnitude. The resultant is inclined by an angle α₂ to \(\overline{A}\)

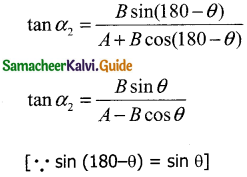
This gives the direction of the resultant. \(\vec{A}\) – \(\vec{B}\)

Question 5.
Write short notes on relative velocity.
Answer:
When two objects A and B are moving with uniform velocities then the velocity of one object A with respect to another object B is called the relative velocity of A with respect to B.

Case 1:
Consider two objects A and B moving with uniform velocities \(\overline{V}\)_A and \(\overline{V}\)_B along straight line in same direction with respect to ground.
The relative velocity of object A with respect to object B is \(\vec{V}\)_AB = \(\vec{V}\)_A – \(\vec{V}\)_B
The relative velocity of object B with respect to object A is \(\vec{V}\)_BA = \(\vec{V}\)_B –\(\overline{V}\)_A
Thus, if two objects are moving it’s the same direction the magnitude of the relative velocity of one object with respect to another is equal to the difference in magnitude of the two velocities.

Case 2:
Consider two objects A and B moving with uniform velocities VA and VB along the same track in the opposite direction

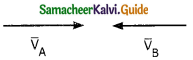
The relative velocity of object A with respect to B is
\(\overline{V}\)_AB = \(\overline{V}\)_A – ( – \(\overline{V}\)_B) = \(\overline{V}\)_A + \(\overline{V}\)_B
The relative velocity of object B with respect to A is
\(\vec{V}\)_BA = – \(\vec{V}\)_B – \(\vec{V}\)_A) = – ( \(\vec{V}\)_A + \(\vec{V}\)_B )
Thus if two objects are moving in opposite directions the magnitude of relative velocity of one object with respect to other is equal to the sum of magnitudes of their velocities.

Case 3:
Consider two objects A&B moving with velocities VA and VB at an angle 0 between their directions, then the relative velocity of A with respect to B

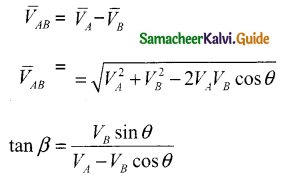
tan θ = (β is the angle between \(\overline{V}\)_AB and VB)

Special cases:
(i) When θ = 0, the bodies move along parallel straight lines in the same direction.
V_AB = (V_A – V_B) in the direction of V_A.
V_BA = (V_B – V_A) in the direction of V_B

(ii) When θ = 180° the bodies move along parallel straight lines in opposite direction.
V_AB = V_A – (- V_B) = (V_A + V_B) in the direction of V_A
V_BA = ( V_B + V_A) in the direction of V_B

(iii) If the two bodies are moving at right angles to each other, then θ = 90°
V_AB = \(\sqrt{V_{A}^{2}+V_{B}^{2}}\)

(iv) Consider a person moving horizontally with velocity \(\vec{V}\)_m Let the rain fall vertically with velocity \(\overline{V}\)_R.

An umbrella is held to avoid the rain.
Then relative velocity \(\overline{V}\)_M of rain with respect to man is

Question 6.
Explain Horizontal projection. Derive the equation for its motion, horizontal range & time of flight.
Answer:

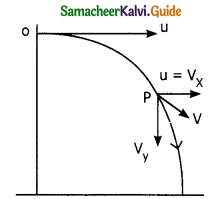
Consider an object thrown horizontally with an initial velocity u, from atop of a tower of height h. The horizontal velocity remains constant throughout its motion and the vertical component of velocity go on increases. The constant acceleration acting along the downward direction is g. The horizontal distance travelled is x(t) = x and the vertical distance travelled is y(t)=y. since the motion is two-dimensional the velocity will have both horizontal (u_x) and vertical (u_y) components.

Motion along horizontal direction:
The particle has zero acceleration along the x-direction and so initial velocity ux remains constant throughout its motion.
The distance travelled by projectile in a time’t’ is given by
x = ut+1/2 at²
x = u_xt → (1)
Motion along vertical direction
Here uy =0, a = g, s = y
S = ut + \(\frac { 1 }{ 2 }\) at²
y = \(\frac { 1 }{ 2 }\) gt² → (2)
from (1) t = x/u_x sub in equation (2)
y = \(\frac { 1 }{ 2 }\) g (x/ux)²
y = k x² Where k = \(\frac{g}{2 u_{x}^{2}}\) .x²
This equation resemble the equation of a parabola. Thus the path followed by the projectile is a parabola.

Expression for time of flight:
The time taken for the projectile to complete its trajectory is called the time of flight.
Let h be the height of the tower or the vertical distance traversed.
Let T be the time of flight w.k. S = ut + 1/2 at²
here s = y = h, u = u_y, t = T, a = g

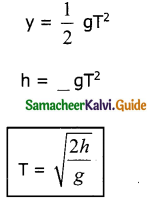
T depends on height of tower or vertical distance & independent of Horizontal velocity.

Expression for Horizontal Range:
The horizontal distance covered by the projectile from the foot of the tower to the point where the projectile hits the ground it called horizontal range.
w. k. t, S = ut + \(\frac { 1 }{ 2 }\) at²
Here,
t = T, a = 0, S = x = R, u = u_x

Hence R ∝ u ∝ & R ∝ \(\frac{1}{\sqrt{g}}\)

Question 7.
Obtain an expression for resultant velocity and the speed of the projectile when it hits the ground in case of a horizontal projection.
Answer:

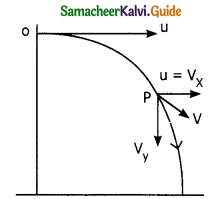
At any instant t, the projectile has velocity components along both the x and y-axis.
The velocity component at any time t along with horizontal component V_x = u → (1)

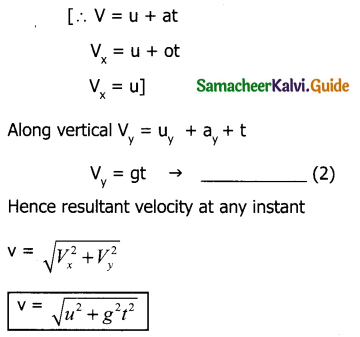
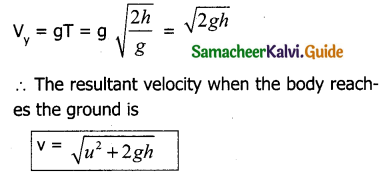
Speed of the projectile when it hits the ground:
When the projectile hits the ground after thrown horizontally from top of tower of height h, the time of flight is T = \(\sqrt{\frac{2 h}{g}}\)
The horizontal component of velocity V_x = u
The vertical component of velocity

Conceptual Questions:

Question 1.
Can a body have a constant speed and still have varying velocity?
Answer:
Yes, a particle in uniform circular motion has a constant speed but varying velocity because of the change in its direction of motion at every point.

Question 2.
When an observer is standing on earth appear the trees and houses appear stationary to him. However, when he is sitting in a moving bus or a train all objects appears to move in a backward direction why?
Answer:
For a stationary observer, the relative velocity of trees and houses is zero. For the observer sitting in the moving train, the relative velocity of houses and trees are negative. So these objects appear to move in the backward direction.

Question 3.
Draw position-time graphs for two objects having zero relative velocity?
Answer:

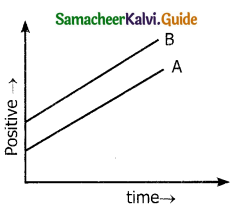
As relative velocity is zero the two bodies A and B have equal velocities. Hence their position-time graphs are parallel straight lines, equally inclined to the time axis.

Question 4.
Can a body be at rest as well as in motion at the same time? Explain.
(OR)
Rest and motion are relative terms. Explain.
Answer:
Yes, the object may be at rest relative to one object and at the same time if maybe in motion relative to another object.

For example, a passenger sitting in a moving train is at rest with respect to his fellow passengers but he is in motion with respect to the objects outside the train. Hence rest and motion are relative terms.

Question 5.
Use integration technique to prove that the distance travelled in-the n^th second of motion is S_th =u + \(\frac { a }{ 2 }\) (2n – 1)
Answer:
By definition of velocity v = \(\frac { ds }{ dt }\)
ds = Vdt = (u + at) dt → (1)
when t = (n – 1) second, let distance travelled = S_n-1
when t = n, second, let distance travelled = S_n

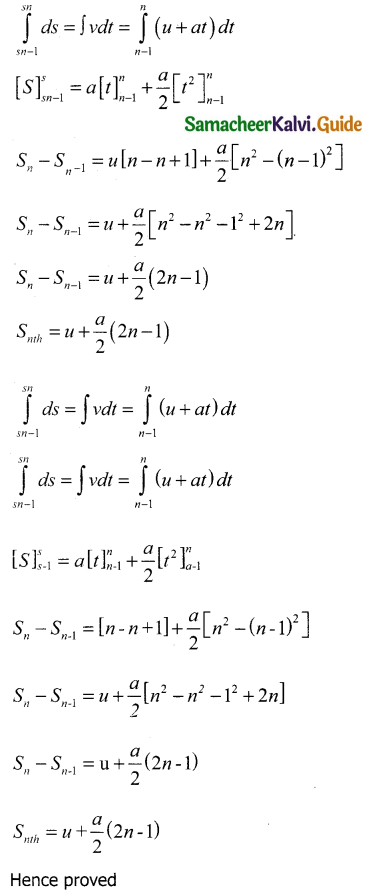
Question 6.
An old lady holding a purse in her hand was crossing the road. She was feeling difficulty in walking. A pickpocket snatched the purse from her and started running away. Can seeing this incident Suresh decided to help that old lady. He informed the police inspector who was standing nearby the inspector chased the pickpocketed and caught hold of him. He collected the purse from the pickpocket and gave back the purse to the old lady.
a) What were the values displayed by Suresh?
b) A police jeep is chasing with a velocity of 45 km/h. A thief in another jeep is moving at 155 km/hr. Police fire a bullet strike the jeep of the thief?
Answer:
The values displayed by Suresh are the presence of mind, helping tendency, and also a sense of social responsibility.
Relative velocity of the bullet with respect to thief’s Jeep = (Vb + Vp)-Vt.
= 180 m/s + 45 km/hr – 155 km/hr
= 180 m/s – 110 x 5/18 m/s
= 180 – 30.5
= 149.5 m/s.

Question 7.
A stone is thrown vertically upwards and then it returns to the thrower. Is it projective?
Answer:
No. It is not a projectile. A projectile should have two-component velocities in two mutually perpendicular directions. But in this case, body has a velocity in only one direction.

Question 8.
Can two non-zero vectors give zero resultant when they multiply with each other?
Answer:
If yes condition for the same. Yes. for example, the cross product of two non-zero vectors will be zero when θ = 0 or θ = 180°.

Question 9.
Justify that a uniform motion is an accelerated motion.
Answer:
In a uniform circular motion, the speed of the body remains the same but the direction of motion changes at every point.

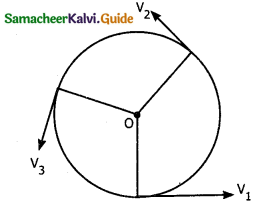
Fig. shows the different velocity vectors at different positions of the particle. At each position, the velocity vector V is perpendicular to the radius vector. Thus the velocity of the body changes continuously due to the continuous change in the direction of motion of the body. As the rate of change is of velocity is acceleration a uniform circular motion is an accelerated motion.

Question 10.
State polygon law of vector addition.
Answer:
If a number of vectors are represented both in magnitude and direction by the sides of an open polygon taken in the same order then their resultant is represented both in magnitude arid direction by the closing side of the polygon taken in the opposite order.