Tamilnadu State Board New Syllabus Samacheer Kalvi 8th Maths Guide Pdf Chapter 5 Geometry Ex 5.5 Text Book Back Questions and Answers, Notes.
Tamilnadu Samacheer Kalvi 8th Maths Solutions Chapter 5 Geometry Ex 5.5
I. Construct the following parallelograms with the given measurements and find their area.
Question 1.
ARTS, AR = 6cm, RT = 5cm and ∠ART = 70°.
Answer:
Given : In the Parallelogram ARTS,
AR = 6 cm, RT = 5 cm, and ∠ART = 70°
Rough Diagram
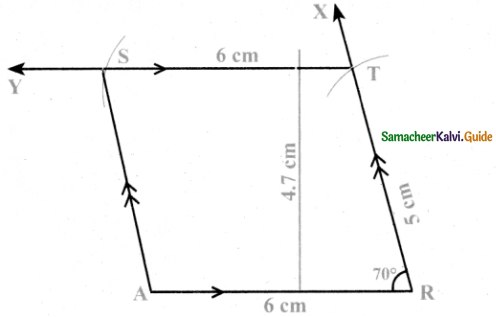
Construction:
Steps:
- Draw a line segment AR = 6 cm.
- Make an angle ∠ART = 70° at R on AR
- With R as centre, draw an arc of radius 5 cm cutting RX at T
- Draw a line TY parallel to AR through T.
- With T as centre, draw an arc of radius 6 cm cutting TY at S. Join AS
- ARTS is the required parallelogram.
Calculation of area:
Area of the parallelogram ARTS = b × h sq. units
= 6 × 4.7 = 28.2 sq.cm
![]()
Question 2.
CAMP, CA = 6cm, AP = 8cm and CP = 5.5cm.
Answer:
Given : In the parallelogram CAMP,
CA = 6 cm, AP = 8 cm, and CP = 5.5cm
Rough Diagram
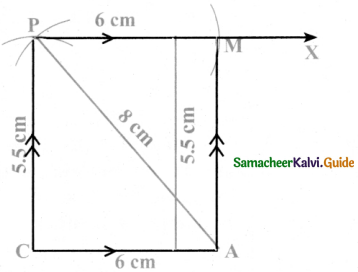
Construction:
Steps:
- Draw a line segment CA = 6 cm.
- With C as centre, draw an arc of length 5.5 cm
- With A as centre, draw an arc of length 8 cm
- Mark the intersecting point of these two arcs as P
- Draw a line PX parallel to CA
- With P as centre draw an arc of radius 6 cm cutting PX at M. Join AM
- CAMP is the required parallelogram.
Calculation of area:
Area of the Parallelogram CAMP = b × h sq. units
= 6 × 5.5 = 33 sq.cm
![]()
Question 3.
EARN, ER = 10cm, AN = 7cm and ∠EOA = 110° where \(\overline{\mathrm{ER}}\) and \(\overline{\mathrm{AN}}\) intersect at O.
Answer:
Given: In the parallelogram EARN,
ER = 10 cm, AN = 7 cm, and LEOA = 1100
Where \(\overline{\mathrm{ER}}\) and \(\overline{\mathrm{AN}}\) intersect at 0
Rough diagram

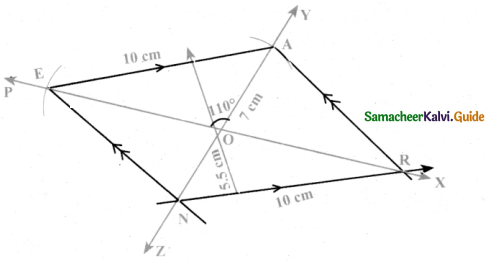
Construction:
Steps:
- Draw a line segment PX. Mark a point O on PX
- Make an angle ∠EOA = 1100 on PX at O
- Draw arcs of radius 3.5 cm with O as centre on either side of PX. Cutting YZ on A and N
- With A as centre, draw an arc of radius 10 cm, cutting PX at E. Join AE
- Draw a line parallel to AE at N cutting PX at R. Join EN and AR
- EARN is the required parallelogram
Calculation of area:
Area of the Parallelogram EARN = b × h sq. units
= 10 × 5.5 = 55 sq.cm
![]()
Question 4.
GAIN, GA = 7.5cm, GI = 9cm and ∠GAI = 100°.
Answer:
Given : In the parallelogram GAIN,
GA = 7.5 cm, GI = 9 cm, and ∠GAI = 100°
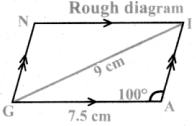
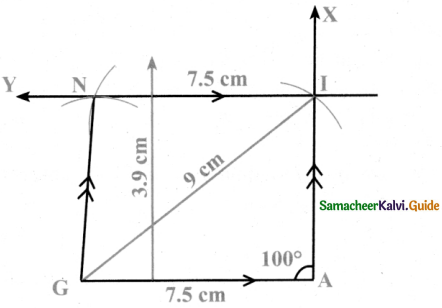
Construction:
Steps:
- Draw a line segment GA = 7.5 cm.
- Make an angle GAI = 100° at A.
- With G as centre, draw an arc of radius 9 cm cutting AX at I. Join GI.
- Draw a line IY parallel to GA through I.
- With I as centre, draw an arc of radius 7.5 cm on IY cutting at N. Join GN
- GAIN is the required parallelogram.
Construction of area:
Area of the Parallelogram GAIN = b × h sq. units
= 7.5 × 39 = 29.25 sq. cm
![]()
II. Construct the following rhombuses with the given measurements and also find their area.
(i) FACE, FA = 6 cm and FC = 8 cm
Answer:
Given FA = 6 cm and FC = 8 cm
Rough Diagram
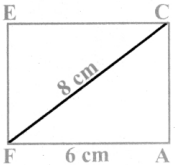

Steps:
- Drawn a line segment FA = 6 cm.
- With F and A as centres, drawn arcs of radii 8 cm and 6 cm respectively and let them cut at C.
- Joined FC and AC.
- With F and C as centres, drawn arcs of radius 6 cm each and let them cut at E.
- Joined FE and EC.
- FACE is the required rhombus.
Calculation of Area :
Area of the rhombus = \(\frac { 1 }{ 2 }\) × d1 × d2 sq.units = \(\frac { 1 }{ 2 }\) × 8 × 9 sq.units = 36 cm
![]()
(ii) CAKE, CA = 5 cm and ∠A = 65°
Answer:
Given CA = 5 cm and ∠A = 65°
Rough Diagram
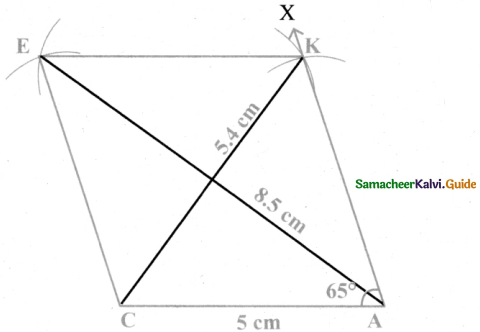
Steps:
- Drawn a line segment CA = 5 cm.
- At A on AC, made ∠CAX = 65°
- With A as centre, drawn arc of radius 5 cm. Let it cut AX at K.
- With K and C as centres, drawn arcs of radius 5 cm each and let them cut at E.
- Joined KE and CE.
- CAKE is the required rhombus.
Calculation of Area:
Area of the rhombus = \(\frac { 1 }{ 2 }\) × d1 × d2 sq.units
= \(\frac { 1 }{ 2 }\) × 54 × 85cm2
= 22.95 cm2
![]()
(iii) LUCK, LC = 7.8 cm and UK = 6 cm
Answer:
Given LC = 7.8 cm and UK = 6 cm
Rough Diagram
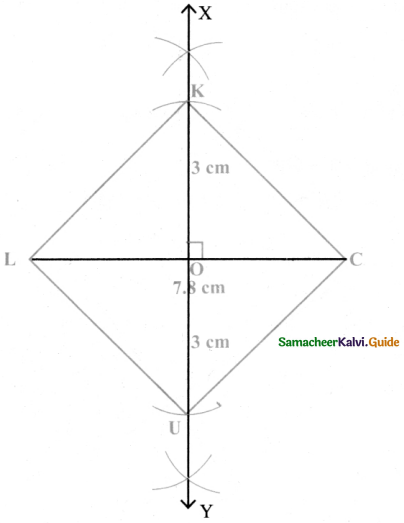
Steps:
- Drawn a line segment LC = 7.8 cm.
- Drawn the perpendicular bisector XY to LC. Let it cut LC at ‘O’
- With O as centres, drawn arc of radius 3 cm on either side of O which cut OX at K and OY at U.
- Joined LU, UC, CK and LK.
- UCK is the required rhombus.
Calculation of Area:
Area of the rhombus = \(\frac { 1 }{ 2 }\) × d1 × d2 sq.units
= \(\frac { 1 }{ 2 }\) × 7.8 × 6 cm2 = 23.4 cm2
![]()
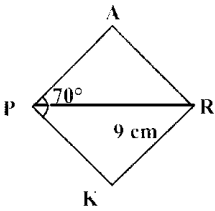
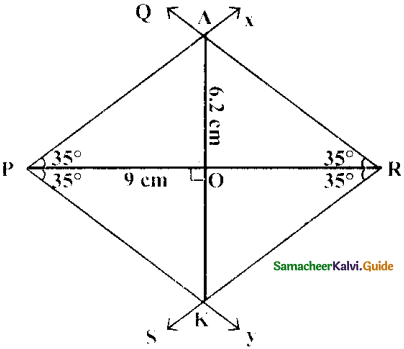
(iv) PARK, PR = 9 cm and ∠P = 70°
Answer:
Given PR = 9 cm and ∠P = 70°
Rough Diagram
Steps:
- Drawn a line segment PR = 9 cm.
- At P, made ∠RPX ∠RPY = 35° on either side of PR.
- At R, made ∠PRQ = ∠PRS = 35° on either side of PR
- Let PX and RQ cut at A and PY and RS at K.
- PARK is the required rhombus
Constructon of Area:
Area of the rhombus = \(\frac { 1 }{ 2 }\) × d1 × d2 sq.units = \(\frac { 1 }{ 2 }\) × 9 × 6.2 cm2
= 27.9 cm2
![]()
III. Construct the following rectangles with the given measurements and also find their area.
(i) HAND,HA = 7cm and AN = 4 cm
Answer:
Given HA = 7cm and AN = 4 cm
Rough Diagram

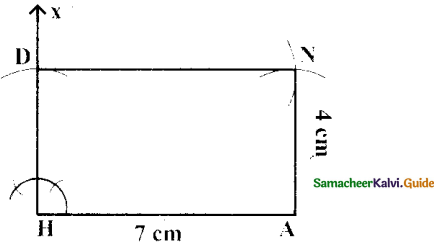
Steps:
- Drawn a line segment HA = 7 cm.
- At H, constructed HX ⊥ HA.
- With H as centre, drawn an arc of radius 4 cm and let it cut at HX at D.
- With A and D as centres, drawn arcs of radii 4 cm and 7 cm respectively and let them cut at N.
- Joined AN and DN.
- HAND is the required rectangle.
calculation of’ area :
Area of the rectangle HAND = l × b sq.units
= 7 × 4 cm2
= 28 cm2
![]()
(ii) LAND, LA = 8cm and AD = 10 cm
Answer:
Given LA = 8cm and AD = 10 cm
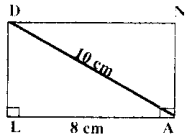
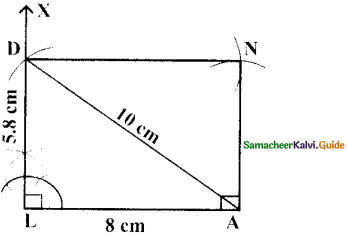
Sleps :
- Drawn a line segment LA = 8 cm.
- At L, constructed LX ⊥ LA.
- With A as centre, drawn an arc of radius 10 cm and let it cut at LX at D.
- With A as centre and LD as radius drawn an arc. Also with D as centre and LA as radius drawn another arc. Let then cut at N.
- Joined DN and AN.
- LAND is the required rectangle.
Calcualtion of arca :
Area of the rectangle LAND = l × b sq.units
= 8 × 5.8 cm2
= 46.4 cm2
![]()
IV. Construct the following squares with the given measurements and also find their area.
(i) EAST, EA = 6.5 cm
Answer:
Given side = 6.5 cm
Rough diagram

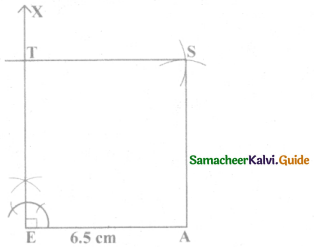
Steps:
- Drawn a line segment EA = 6.5 cm.
- At E, constructed EX⊥ EA.
- With E as centre, drawn an arc of radius 6.5 cm and let it cut EX at T.
- With A and T as centre drawn an arc of radius 6.5 cm each and let them cut at S.
- Joined TS and AS.
- EAST is the required square.
Calcualtion of Area:
Area of the square EAST = a2 sq.units
= 6.5 × 6.5 cm2
= 42.25 cm2
![]()
(ii) WEST, WS = 7.5 cm
Answer:
Given diagonal = 7.5 cm
Rough Diagram
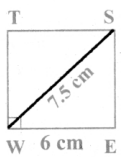
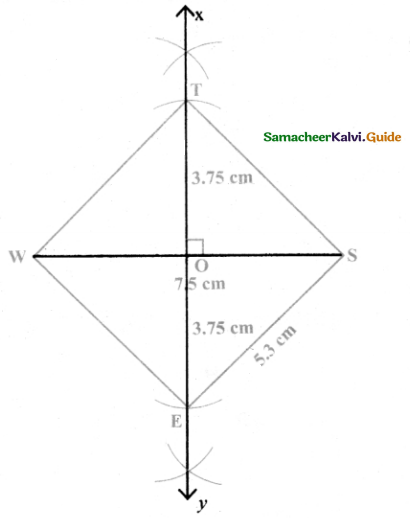
Steps:
- Drawn a line segment WS = 7.5 cm.
- Drawn the perpendicular bisector XY to WS. Let it bisect BS at O.
- With O as centre, drawn an arc of radius 3.7 cm on either side of O which cut OX at T and OY at E
- Joined BE, ES, ST and BT.
- WEST is the required square.
Calculation of Area:
Area of the square WEST = a2 sq.units
= 5.3 × 53 cm2
= 28.09 cm2.