Samacheer Kalvi 8th Maths Guide Chapter 2 Measurements Ex 2.4

Tamilnadu State Board New Syllabus Samacheer Kalvi 8th Maths Guide Pdf Chapter 2 Measurements Ex 2.4 Text Book Back Questions and Answers, Notes.

Tamilnadu Samacheer Kalvi 8th Maths Solutions Chapter 2 Measurements Ex 2.4

Question 1.
Two gates are fitted at the entrance of a library. To open the gates easily, a wheel is fixed at 6 feet distance from the wall to which the gate is fixed. If one of the gates is opened to 90°, find the distance moved by the wheel (π = 3.14).

Amswer:
Let A be the position of the wall AC be the gate in initial position and AB be position when it is moved 90°.
Now the arc length BC gives the distance moved by the wheel.

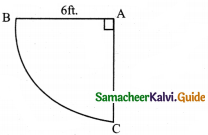
Length of the arc = \(\frac{\theta}{360^{\circ}}\) × 2πr units
= \(\frac{90^{\circ}}{360^{\circ}}\) × 2 × 3.14 × 6 feets
= 3.14 × 3 feets
= 9.42 feets
∴ Distance moved by the wheel = 9.42 feets.

Question 2.
With his usual speed, if a person covers a circular track of radius 150 m in 9 minutes, find the distance that he covers in 3 minutes (π = 3.14).
Answer:
Radius of the circular track = 150m
Distance covers in 9 minutes = Perimeter of the circle = 2 × π × r units
Distance covered in 9 min = 2 × 3.14 × 150m
Distance covered in 1 min = \(\frac{2 \times 3.14 \times 150}{9} \mathrm{m}\)

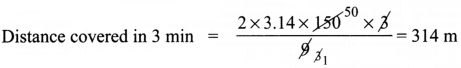
Distance he covers in 3min = 314m

Question 3.
Find the area of the house drawing given in the figure.

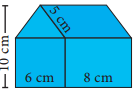
Answer:
Area of the house = Area of a square of side 6 cm + Area of a rectangle with l = 8cm, b = 6 cm + Area of a ∆ with b = 6 cm and h = 4 cm + Area of a parallelogram with b = 8 cm, h = 4 cm
= (side × side) + (l × b) + (\(\frac { 1 }{ 2 }\) × b × h) + bh cm²
= (6 × 6) + (8 × 6) + (\(\frac { 1 }{ 2 }\) × 6 × 4) + (8 × 4) cm²
= 36 + 48 + 12 + 32 cm²
= 128 cm²
Required Area = 128 cm²

Question 4.
Draw the top, front and side view of the following solid shapes
(i)

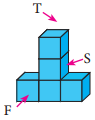
Answer:
(a) Top view

(b) Front view

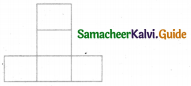
(c) Side view

(ii)

Answer:
(a) Top view

(b) Front view

(c) Side view

Challenging Problems

Question 5.
Guna has fixed a single door of width 3 feet in his room where as Nathan has fixed a double door, each of width 1 \(\frac { 1 }{ 2 }\) feet in his room. From the closed position, if each of the single and double doors can open up to 120°, whose door takes a minimum area?
Answer:
Width of the door that Guna fixed = 3 feet.
When the door is open the radius of the sector = 3 feet

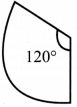
Angle covered = 120°
∴ Area required to open the door

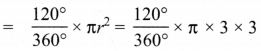
= 3π feet²

(b) Width of the double doors that Nathan fixed = 1 \(\frac { 1 }{ 2 }\) feet.
Angle described to open = 120°
Area required to open = 2 × Area of the sector
z
= \(\frac { 1 }{ 2 }\) (3π) feet²
∴ The double door requires the minimum area.

Question 6.
In a rectangular field which measures 15 m x 8m, cows are tied with a rope of length 3m at four corners of the field and also at the centre. Find the area of the field where none of the cow can graze. (π = 3.14).
Answer:
Area of the field where none of the cow can graze = Area of the rectangle – [Area of 4 quadrant circles] – Area of a circle

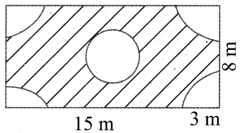
Area of the rectangle = l × b units²
= 15 × 8m² = 120m²
Area of 4 quadrant circles = 4 × \(\frac { 1 }{ 4 }\) πr² units
Radius ofthe circle = 3m
Area of 4 quadrant circles = 4 × \(\frac { 1 }{ 4 }\) × 3.14 × 3 × 3 = 28.26 m²
Area of the circle at the middle = πr² units
= 3.14 × 3 × 3m² = 28.26m²
∴ Area where none of the cows can graze
= [120 – 28.26 – 28.26]m² = 120 – 56.52 m² = 63.48 m²

Question 7.
Three identical coins, each of diameter 6 cm are placed as shown. Find the area of the shaded region between the coins. (π = 3.14) (√3 = 1.732)

Answer:
Given diameter of the coins = 6 cm
∴ Radius of the coins = \(\frac { 6 }{ 2 }\) = 3 cm
Area of the shaded region = Area of equilateral triangle – Area of 3 sectors of angle 60°

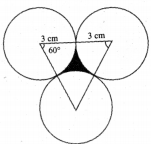
Area of the equilateral triangle = \(\frac{\sqrt{3}}{4}\) a² units² = \(\frac{\sqrt{3}}{4}\) × 6 × 6 cm²
= \(\frac{1.732}{4}\) × 6 × 6 cm² = 15.588 cm²
Area of 3 sectors = 3 × \(\frac{\theta}{360^{\circ}}\) × πr² sq.units
= 3 × \(\frac{60^{\circ}}{360^{\circ}}\) × 3.14 × 3 × 3 cm² = 1.458 cm²
∴ Area of the shaded region = 15.588 – 14.13 cm² = 1.458 cm²
Required area = 1.458 cm² (approximately)

Question 8.
Using Euler’s formula, find the unknowns.

Answer:
Euler’s formula is given by F + V – E = 2
(i) V = 6, E = 14
By Euler’s formula = F + 6 – 14 = 2
F = 2 + 14 – 6
F = 10

(ii) F = 8,E = 10
By Euler’s formula = 8 + V – 10 = 2
V = 2 – 8 + 10
V = 4

(iii) F = 20, V = 10
By Euler’s formula = 20 + 10 — E = 2
30 – E = 2
E = 30 – 2.
E = 28
Tabulating the required unknowns